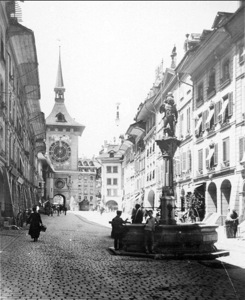

























































Ten Years to Relativity
Below is a chapter from the book Kinematics: The Lost Origins of Einstein’s Relativity (Johns Hopkins University Press, 2009). Contrary to nearly all accounts of the history of special relativity, this one proceeds chronologically and avoids common mistakes, speculations and exaggerations. In the very popular field of the history of special relativity, I’m glad to say, no other work uses so many sources to reconstruct the development of Einstein’s thoughts. I made the text understandable to any interested readers, but the book’s price was too high (as I complained to the publishers; academic presses often overprice books), so to help make it available, here’s a chapter. I’ve added the most extensive collection of historic images of people, places, books, etc., from this period of Einstein’s life. Some are links to other websites, as the cursor will show. Clicking on book images leads to the work itself. For English translations, if available, click on the caption beneath the book image. Page numbers correspond to the printed book (though in some cases I linked orphan sentences to the paragraph in the adjacent page). For original source citations (footnotes) please consult the printed book, I encourage you to actually look it up; books wait for their ideal reader!

Portrait, late 1902, by Emil Vollenweider at
Postgasse 68, Bern. Soon, their civil wedding
was witnessed by Solovine and Habicht.



Habicht, Solovine, and Einstein: “The Olympia Academy,” portrait by photographer Emil Vollenweider, in the summer of 1905.
Mileva Maric, Hans Albert, and Albert Einstein, in 1905.



Einstein at the Swiss Federal Office of Intellectual Property, Bern, in 1904.





Michele Besso, 1898.



Einstein’s early mentor and friend, Max Talmud.
Marcel Grossmann, student of mathematics.
Mileva Maric, Albert Einstein and their
son Hans Albert, at their apartment on
49 Kramgasse in 1904.



Albert Einstein, ca. 1895.
The Zurich Polytechnikum
Mileva Maric, 1896.



Conrad Habicht

Moritz Solovine

Prof. Hermann Minkowski, mathematician.
At the Poly, Einstein often skipped the
mathematical seminars; so years later,
Minkowski recalled him as “a lazy dog.”
Michelson and Morley’s experiment to detect Earth’s motion relative to the ether, 1887; photo, and reflected paths of light.
A corner of the ceiling of the bedroom where Einstein slept in early May 1905.






Front door lock to Einstein’s apartment.



Steps of the staircase winding up to the second apartment at 49 Kramgasse.


Spring morning view of Kramgasse street.
As Einstein walked downstairs from his
apartment and turned left to walk to work,
this is the view that he saw every day.

Michele Besso and his wife Anna.



49 Kramgasse street building; Einstein lived in apartment 2, on the third floor.

Conrad Habicht, smoking a cigar
as usual, in 1905.

Chapter 6
Discovery and Invention:
Conceptual Origins of Einstein’s Relativity
A new theory of kinematics appeared in September 1905 in a paper on electrodynamics in the journal Annalen der Physik. The author of “On the Electrodynamics of Moving Bodies,” Albert Einstein, was not even a lecturer at any university. In the words of John Stachel:
“A twenty-six year old patent expert (third class), largely self-taught in physics, who had never seen a theoretical physicist (as he later put it), let alone worked with one, author of several competent but not particularly distinguished papers, Einstein produced four extraordinary works in the year 1905, only one of which (not the relativity paper) seemed obviously related to the earlier papers. These works exerted the most profound influence on the development of physics in the 20th Century. How did Einstein do it?”1
Scarcely any contemporary documents show how he formulated his kinematic theory. Few extant traces exist of his speculations and analyses prior to his 1905 paper, none of his rough drafts. Few contemporary letters mention some of his ideas. Nonetheless, several historians have thoughtfully and carefully labored to reconstruct at least some aspects of Einstein’s thoughts, piecemeal, and with considerable success. But despite many varied efforts, “no general consensus has emerged” on the origins of Einstein’s theory.2
bottom of p.209

What follows isn’t a history of electrodynamics, it’s a biographical account of how Einstein made a new kinematics. Few contemporary documents track his thoughts on that field since, unlike electrodynamics and optics, it was not a main area of his inquiry. However, many later accounts and letters bear significant traces pertaining to the roots of his ideas. One must carefully organize the findings, and the task of reconstruction is often like that of a paleontologist, trying to construe a life on the basis of just a few deteriorated bones. It’s surprising how much one can learn from even little bits of evidence.
Yet the excess of speculation that sometimes gets incorporated into the reconstruction is disconcerting. Biographers are often moved by literary aspirations and psychological hypotheses. These tendencies often lead them to concoct plausible and lifelike accounts held together but by the glue of the imagination. But plausible fiction and history are distinct. Conjectures do sometimes lead to previously unknown documentary evidence; but lacking such newfound evidence, historical hypotheses are just speculations. Too often people succumb to the impulse to say plenty more than the available evidence warrants. Conjectures are often far less imaginative than they seem; what they often belie is a laziness to imagine additional plausible explanations that, in turn, belittle the first as but merely one hypothesis among many.
So reader beware. Accounts of the origins of special relativity have often been thick in conjectures and thin on facts. People tell stories, and fiction sometimes inspires and incites productivity, so all good stories are welcome. It’s often fine not to dismiss histories tainted with fiction, but well to ponder the hypotheses there advanced. Yet what follows is not a good story; if you want one, there are plenty at the bookstore.
Adding to the evidence that predates 1905, there exist many retrospective statements pertaining to the roots of special relativity, which were later voiced by Einstein and his peers over decades. Due to his success, he was interviewed by physicists, reporters, biographers, psychologists, and historians. Friends, relatives, and coworkers inquired about his creativity. By systematically bringing together this wealth of bits of evidence, a remarkably detailed picture emerges. Too often, writers have neglected chronology and have overemphasized selected bits of evidence. The following narrative is organized chronologically, to the extent that it seemed possible, and it’s built from documentary evidence.
bottom of p.210



Speculations of a College Student
In the late 1880s, Heinrich Hertz demonstrated experimentally that invisible electromagnetic effects traverse space like waves in a medium. His findings fulfilled a main prediction of Maxwell’s theory of electromagnetism. The technological possibilities were extraordinary: messages could hence be transmitted with invisible signals without using wires, across considerable distances. Such signals provided further compelling evidence that vacuum space is not empty but is replete with a subtle invisible medium, known then as the ether. Hertz’s experiments quickly became widely known among physicists and the public.
Like many others, Albert Einstein was a young lad who became interested in questions about electromagnetic waves in the ether. His father and uncle owned and operated a business that developed and distributed electrical technologies, such as lamps and dynamos. As a boy, Albert had been fascinated by magnetism. In 1895, the sixteen-year old wrote an essay pondering “the state of the ether in the magnetic field.”3 Having heard about “Hertz’s wonderful experiments,” Albert proposed to investigate how a magnet affects the structure of the ether. He argued that there should be variations in the speed of an electromagnetic wave, such as a ray of light, passing through a magnetic field. By studying such variations, he hoped, one might measure the deformations of the ether. Such speculations about variations in the motion of light relative to bodies in the ether arose occasionally, even in magazines about science and technology.
Thus the young Einstein began to cultivate an interest in questions about the propagation of light. As we know, the concept of the relativity of motion had been around for a long time. How did it begin to gain importance for him? In 1895, he was preparing to take entrance examinations to a technical school at Zurich, Switzerland. As reported by his sister Maja many years later, one of the sixteen-year-old’s books was a textbook by Jules Violle on mechanics.4 Violle based his treatment of dynamics on
bottom of p.211


the “principle of relative motions” together with the principle of inertia.5 The date when Einstein studied Violle, 1895, coincides with his later claim that he pondered the problems of the relative motion of light for ten years prior to 1905.6
Still, we can hardly infer that Einstein’s lifelong interest in relative motion originated from Violle, at least not from that textbook alone. Other experiences could also have contributed to his interest. For example, already at the age of thirteen, Albert had a favorite philosopher.7 Immanuel Kant had famously advocated, in 1758, what he called “new” concepts of motion and rest, in opposition to Newton’s views on absolute motion, by arguing that one should never talk about the motion of any given body without first specifying relative to what other body it is said to move. According to a third-hand account, the young Albert “especially liked the views of Immanuel Kant and, as he told a friend later, it was through Kant’s philosophy that he began to question the truth of generally held beliefs.”8 Reportedly, Albert and Max Talmud discussed “at great length Immanuel Kant’s observations concerning the objective and subjective attitude toward knowledge.”9 In any case, we do not know whether at an early age he studied or particularly cared for Kant’s views on motion; apparently Einstein left no comments on the matter. The point is that along with Violle’s textbook other sources were at hand.
bottom of p.212

Alongside ideas on relative motion, ideas on absolute motion also abounded. The young Albert could read even the Preface of the Mathematical Principles of Natural Philosophy and learn that Newton had composed it mainly to advance the knowledge of true motions rather than of motions merely apparent. In the 1890s most physicists believed that all uniform rectilinear motion is relative, at least in the sense that absolute motion could not be detected by mechanical means; but nevertheless, they also believed that true absolute motion existed, relative to space itself, even if it could not be detected. This belief did not seem to be unverifiable. Rather, owing to electromagnetic theory, physicists thought they had at hand some theoretically plausible ways to establish a kind of seemingly absolute motion. Experiments had demonstrated that light seems to propagate like waves in a medium. Many experiments suggested that this medium, the ether, could not be moved. If the ether were really fixed in space then perhaps some experiment would detect the motion of bodies relative to it.
Later in life Einstein recalled that at the age of sixteen he became puzzled by the relationship between light and bodies in motion. He was a student at a school in Aarau, when he conceived a “childish thought-experiment,” he asked himself: “When one chases a lightwave at the speed of light, one would have a time-independent wavefield. But yet there seemed to exist no such thing!”10 So really, “the youth of sixteen pondered: what would happen if a man should try to capture a ray of light?”11 A psychologist interviewed him in 1916, and noted:
“The process started in a way that was not very clear, and is therefore difficult to describe—in a certain state of being puzzled. First came such questions as: What if one were to run after a ray of light? What if one were riding on the beam? If one were to run after a ray of light as it travels, would its velocity thereby be decreased? If one were to run fast enough, would it no longer move at all?”12
bottom of p.213



Another subject of interest was Maxwell’s theory of electricity and magnetism. It was the chief means by which physicists investigated questions about light and the ether. Albert was disappointed that the teachers at the Poly did not offer lessons on Maxwell’s theory.31 But he proceeded to study it thoroughly on his own, with the help of books such as August Föppl’s popular textbook and similar works.32 Contrary to his early expectations, he learned that physicists believed that the behavior of light should vary depending on the state of motion of the observer. This conviction was based on Augustin Fresnel’s wave theory of light, along with Maxwell’s theory of electromagnetic fields. In Maxwell’s theory the speed of electromagnetic radiation, such as light, in a vacuum, was as a constant. But if an observer moved relative to the ether then that observer should be able to detect relative variations in the speed of light. Thus Einstein encountered, “in his second year of college, the problem of light, ether and the Earth’s movement. This problem never left him.”33 As the Earth moves through the ether, variations in the speed of light rays should be detectable. So in 1898 Einstein began to devote much thinking to investigate the question of light and relative motion. Years later he recalled that from 1898 until 1905, that problem was essentially “my life for over seven years and that this was the main thing.”34
Following Maxwell, most physicists believed that the equations of electromagnetic theory implied that as bodies move rectilinearly through the luminiferous ether, relative to such bodies there would exist certain asymmetries in the propagation of light, that is, if the ether were absolutely “at rest” in space. Hence, even if perhaps one were never able to identify absolute motions, that is, in space itself, one might be able to identify the motion of bodies relative to the ether. Like many physicists, Albert became increasingly interested in this question, though still a student. It was a subtle issue. Föppl, for instance, appreciated Mach’s dislike for unobservable hypothetical entities,
bottom of p.218


Albert also met Michele Angelo Besso, at a house where various people gathered on Saturday afternoons to play music. Six years older than Albert, he also had broad interests in physics and philosophy. He had graduated from mechanical engineering at the Poly in 1895, and in the Fall of 1896 he had started working at a factory of electrical machines in Winterthur.
Michele and Albert discussed various subjects, including the then-popular questions about the existence of atoms and the ether.18 In this connection, in 1897, Michele recommended to Albert that he read Ernst Mach’s critical book on the history and concepts of mechanics.19 Michele had turned to Mach’s writings while he was a student at the Poly, under the suggestion of Aurel Stodola, Professor of mechanical engineering.20 Mach was skeptical of the existence of allegedly invisible entities that could not be detected experimentally. He was particularly critical of the concept of atoms, as well as the notions of absolute space and absolute time. Mach argued that instead of referring motions to absolute space, motions should be established only relative to material systems. Absolute motion had no physical significance, but motions relative to the universe as a whole could perhaps be ascertained by reference to the ether, the stars, or all the bodies in the universe.
Albert was interested in the conceptual underpinnings of physics. Kant had argued that certain organizational categories of the mind are unchangeable; such as the notion of causality. Yet he had also argued that there are others, “concepts,” that can be constructed from experience. Albert learned that “whatever in knowledge is of empirical origin is never certain.”21 He developed the opinion that laws of physics “owe their origin to the inventive faculty of the human mind. It was this very point that Einstein esteemed so highly in Kant’s work. Kant’s principal point was that the general laws of science contain not only the result of experience, but also an element provided by human reason.”22 Reportedly, his early interest in Kant’s outlook encouraged him “to work out his own original theories.”23
bottom of p.215

Thanks to philosophers such as Kant and Arthur Schopenhauer, Einstein could increasingly acknowledge the imperfections of general concepts, how they are constructed and rooted in perception.24 Schopenhauer, for example, argued that, “All concepts, all things that are thought, are indeed only abstractions, and consequently partial representations from perception, and have arisen merely through our thinking something away.”25 Likewise, in the evenings, while at the Poly, Einstein began to study the works of Ludwig Boltzmann, one who argued that “To construct thought pictures we constantly need designations for what is common to various groups of phenomena, thought-pictures or intellectual operations; we call such designations concepts...”26
Once his first academic year at the Poly ended, having studied mainly courses on mathematics, Einstein received good grades. Likewise, Mileva Maric successfully completed her coursework, and she then left the Poly to audit courses at Heidelberg. Einstein was disappointed but initiated a lasting correspondence with her. Still, in the Fall of 1897, Einstein
bottom of p.216


continued to spend time with his friend Marcel Grossmann and other classmates who also studied mathematics and physics. One classmate, Walter Leich, recollected that in the daily recesses:
“It was our habit to walk back and forth in the long hall off of the lecture rooms. We carried on lively discussions about subjects we were studying and about things in general.
“The most animated talker was the alert and capable student Marcel Grossmann, the most reserved Einstein. These two young men became close friends and were much together away from school. …
“Einstein was a pleasant, gentle, soft spoken person. In class-room tests he acquitted himself as well as but no better than others in the class. … His spirit of independence asserted itself one day in class when the professor mentioned a mild disciplinary measure just taken by the school authorities. Einstein for once raised his voice energetically to the effect that students at the Polytechnic School should not be subjected to strict regulations.”27
Einstein enjoyed the independence that the Poly allowed. Attendance in many seminars was not mandatory. So he pursued his interests in physics independently.
At the time, Einstein was very impressed by “the achievements of mechanics in areas which apparently had nothing to do with mechanics,” such as: “the mechanical theory of light, which conceived of light as the wave-motion of a quasi-rigid elastic ether…”28 Above all, he was impressed by the kinetic theory of gases. Yet his faith in mechanics was shaken by Mach’s skeptical attitude towards its foundations; Mach’s book “exercised a profound influence upon me in this regard, while I was a student.”29 One of the major questions discussed in those days was: Do molecules exist?30 Einstein learned that leading scientists such as Mach and Wilhelm Ostwald had stated boldly that they did not believe in the existence of molecules and atoms. His older friend, Michele Besso, also introduced him to Mach’s work on the theory of heat, which Einstein enjoyed as well.
bottom of p.217


Hence, “The problem that occupied him was the optics of moving bodies, or, more exactly, the emission of light from bodies that move relative to the ether.”13 It seemed paradoxical, if one could move fast enough, one would see a weird phenomenon: stationary light. He knew no such thing to exist so he expected instead that “from the standpoint of such an observer” everything should transpire exactly as for an observer at rest.14 Motion might be relative, there would be no way to tell, by looking at the behavior of light, whether one is absolutely moving or at rest. Thus Einstein became curious about “problems in the optics of moving bodies.”15
Einstein entered the Polytechnikum at Zurich in the fall of 1896, at the age of seventeen and a half, six months shy of the required minimum age. Other students, such as Louis Kollros, noted that he was the youngest.16 At first, Albert was lonely and quiet. But he made a few friends with whom to discuss his interests. Among them was the lively Marcel Grossmann, a diligent student of mathematics. The two would often meet at the Café Metropol to talk.17 Another new acquaintance was Mileva Maric, a reserved student entering the physics Section too in 1896.
bottom of p.214


and thus he tried to abstain from referring to the ether. In addition to Föppl’s book, Einstein also read works by Gustav Kirchhoff, Hermann von Helmholtz, and Hertz, all with “a veritable mania for reading, day and night.”35
By mid-April of 1898, Mileva Maric was back in Zurich, to enroll again in the Poly for the summer semester, which was just beginning. The nineteen-year-old Albert was glad she returned. At that time, he began to analyze Maxwell’s theory intensively by studying Paul Drude’s Physics of the Aether of 1894.36 In that book Drude sought an economical formulation of electromagnetic theory: he avoided any attempts to formulate mechanical models of the ether, he avoided the concept of ether velocity, and even suggested ascribing to space itself the properties required to account for electromagnetic phenomena.
The Poly gave students leeway to pursue their interests. Albert often skipped the mathematical lectures, preferring instead to spend most of his time in the physical laboratory. He was “fascinated by the direct contact with experience.”37 In the winter semester of 1898, he began courses on electricity and electrical technologies with Professor H. F. Weber. He learned much from Weber, even about the atomistic nature of electrical and thermal phenomena. He enjoyed being at Weber’s laboratory, one of the best-equipped in Europe. Yet Einstein disliked another Professor, Jean Pernet, as incompetent and insane, which earned him a reprimand.
On the way home after working in the lab, Einstein and company sometimes talked about ideas in physics. One older classmate, Margarete von Uexküll, who boarded in the same house as Mileva, later recalled Einstein once talking about gravity, mass, and time. According to her, he argued as they walked: “You can well imagine the universe as a sphere filled with any mass, the starry world, gases, ether or however one may call it. Consider now this mass gone, then also there would exist no more time.” She listened, but felt none the wiser.38
bottom of p.219


After school-hours, Einstein continued his independent studies of theoretical physics: “This extended private study was simply the continuation of earlier habit; and in this Mileva Maric took part.”39 With Mileva, Albert reread some of the works he had already read.40 To her peers, and even her close friends, Mileva appeared to be too serious and quiet, but good-natured and very intelligent.41 Reportedly, “She and Einstein found a common interest in their passion for the study of the great physicists, and they spent a great deal of time together.”42 Thus Albert had “found a partner in these studies who was working in a similar direction.”43 Although she was four years older than him, he regarded her, to some extent, as “my ‘student’.”44 Einstein was interested not only in the ideas of scientists, but also in aspects of their biographies.45 According to one writer, one of the books that they read was a biography of Newton:
“Both Albert and Mileva thought that to understand a man’s work they ought to know something about the man himself. After they had finished reading a biography of Sir Isaac Newton, the seventeenth-century English mathematician, physicist, and philosopher, Albert asked Mileva, “Have you ever thought that Newton might be wrong in some of his conclusions?” Mileva stared at him as if he had questioned the unquestionable. “Wrong about what?” she asked. “Well, it seems to me that many times Newton doesn’t give proofs for what he calls laws. He just says, ‘This is so.’ Couldn’t there be forces about which he knew nothing?” “You never simply accept a theorem, a mathematical solution, or a physical law, do you?” asked Mileva. “Always you ask why, as if nothing ever had been said on the subject.” Albert grinned but did not try to explain that for him study became a pleasure only when it led to understanding.”46
bottom of p.220


Reportedly, Einstein had been convinced by his readings of Kant “that man-found laws of science which stood up to test derived from the inventive element of human reason as well as from experience.”47 Also, Einstein now shared Mach’s critical-historical skepticism towards the foundations of mechanics.48 He appreciated that which could be demonstrated experimentally more than that which was postulated as fundamental assumptions.
The young Einstein believed that there ought to be a way of detecting motion relative to the ether experimentally. Reportedly, in those days “His entire concern was always with the visible processes of physics.”49 He searched in the physics literature but found no experiment, not “any fact that demonstrates this current of ether, in the physics literature, even in the smallest way.”50 Thus he decided to pursue the problem:
“So, I thought, I wanted to try to demonstrate this ether current against the Earth, in other words, the motion of the Earth. Back then, when I raised this problem in my mind, I did not doubt at all the existence of the ether and the motion of the Earth. Therefore, I sought to examine the difference of amount of heat arising from using two thermoelectric couples, predicting that there should be a difference in the energy between the orientation of the Earth’s motion, and the opposite direction, by appropriately reflecting with a mirror the light from a single source.”51
bottom of p.221


He thought that by measuring the heat or pressure generated by light rays propagating in different directions, one might be able to detect the motion of the Earth through the universal medium. Hence Einstein entertained the idea of designing such an experiment, but he “did not formulate this experiment with thorough clarity.”52 Meanwhile, practicing physicists had designed and performed many precise experiments with the same aim, but no such effects were found systematically. (Einstein did not then know of such attempts.)53 Accordingly, it seems that his Professors at the Poly were not interested in carrying out his experiment, or giving him the opportunity, so it remained a mere idea.54
In any case, basic electromagnetic experiments exhibited seemingly perfectly symmetric effects of relative motion. One in particular was very well known, even at an elementary level, because it exhibited a dynamic connection between electricity and magnetism. It was an old experiment. In 1831 Michael Faraday had discovered the effect: that the relative motion between a magnet and a conducting wire induces an electric current in the wire, and the effect is the same irrespective of whether one moves the wire or the magnet. For Einstein this was a problem because Maxwell’s theory, as commonly interpreted, provided different explanations for this effect, depending on whether the magnet or the wire moves, whereas, the observed effect is identical. In both cases, the same relative motion induces an identical electrical current in the wire. Historians have argued that Einstein may have drawn this case of electromagnetic induction from Föppl’s textbook of 1894.55 Other writers did not describe induction in terms of problematic asymmetries and the violation of an “axiom of kinematics.”56
During the course of his formal education, Einstein learned about kinematics from a variety of sources. At the Poly he took a course on the mechanics of rigid-body motion in which the Professor, Hermann Minkowski, explained: “One speaks of kinematics when one investigates only the geometrical possibilities of motion, where only the concepts of space and time
bottom of p.222


are considered.”57 Minkowski, a mathematician, had been an enthusiastic follower of Hertz in his attempt to eliminate the concept of force from the foundations of physics. While studying at the Poly, Einstein began to read Hertz’s Principles of Mechanics of 1894 with interest, it was a popular book. In it, Hertz complained about the obscurities in the foundations of mechanics, and he made occasional use of the term “kinematics.”58 Hertz even commented: “Why is it that one never asks what is the nature of velocity?” Yet he focused mainly on critically revising the concept of force. Einstein’s recurring thoughts involved the more specific question: “What is ‘the velocity of light’?”59 He reportedly stopped reading Hertz’s book at the point where Hertz replaced all physical forces with purely mathematical connections between particles.60 Rather than focus on kinematics, Einstein was much more interested in others subjects, especially Maxwell’s electromagnetic theory.
Einstein discussed the questions of relative motion in connection with electromagnetic theory, with Mileva Maric, who by then was his intimate friend. A good friend later recalled how one day Einstein talked about Maric: “I often work with her. She understands nearly as much mathematics as I myself; for me it is a prize that I’ve had the opportunity to get to know her. She is not downright beautiful, but she is very clever.”61 In contradistinction, a later friend and colleague of Einstein noted that Maric “was taciturn and reticent,” but that “Einstein in his zeal for his studies hardly noticed it.”62
bottom of p.223


In the summer of 1899, while studying Hertz’s work on the propagation of electrical actions, Albert wrote to Mileva that he was increasingly convinced that the theory of electrodynamics of moving bodies did not correspond to reality. He complained that it involved “the name ‘ether’” as a medium that was described as being in motion (relative to the Earth), but yet such motion had no physical meaning, no way of being detected.63 Perhaps it would be possible to formulate an electrodynamics of moving bodies that did not refer motion to the ether. Einstein hoped to reformulate electrodynamics as the science of “moving electricities and magnetisms” in empty space. He had also studied the papers of Hermann von Helmholtz who had argued that electricity consisted of free particles. Their existence, by the late 1890s had been recognized by many physicists, on experimental grounds.
By September 1899 Einstein seems to have read a work by Wilhelm Wien that briefly summarized thirteen experiments that had failed to detect the motion of the Earth relative to the ether.64 Among these experiments were the attempts by Albert Abraham Michelson to detect the “ether wind” by comparing the interference effects of beams of light shot in orthogonal directions and brought back together by mirrors. Michelson’s experiment was becoming increasingly notorious, because it had shown, to an unprecedented degree of precision, that the presumed relative motion of the ether through the Earth generated no detectable effect, contrary to expectations. One might begin to suspect that rectilinear motion relative to the ether could hardly be detected. In hindsight, regarding Michelson’s result, Einstein once commented: “I guess I just took it for granted that it was true.”65
But although such experiments had failed, there existed at least slight indications that the ether seemed to be partially affected by the motion of matter. In particular, a famous experiment reported by Hippolyte Fizeau already in 1851 had shown that light behaves oddly in moving water. When traversing moving water, the speed of light seemed affected by the water’s motion. It was as though the ether itself were dragged along by the moving water, but only very slightly so. If the ether were dragged completely one would expect that the total speed of light would be the speed of light in water, c/n, plus the water’s speed, w. Instead, the observations seemed to give a total speed of c/n + kw, where k < 1. The term k, known as the Fresnel drag coefficient,
bottom of p.224



If theory hardly settled these matters, one might turn to experiment. In September of 1899 Albert wrote to Mileva that he “had a good idea for investigating the way in which a body’s relative motion affects the velocity of the propagation of light in transparent bodies. I even came up with a theory about it that seems quite plausible to me.”71 Again, he did not carry out any such experiment. Later that month he told Mileva that “I also wrote a to Professor Wien in Aachen about my paper on the relative motion of the luminiferous ether against ponderable matter, the paper which the ‘boss’ handled in such an offhanded fashion.”72 Albert had drafted a paper concerning the ether motion but, apparently, Professor Weber had been dismissive, so he hoped that Wien would find it interesting. But it seems that Wien did not reply. Regardless, Albert found a different kind of success, as Mileva became his girlfriend.
Contrary to standard practice, Einstein himself chose the subject for his diploma paper (and he also chose one for Mileva).73 Then he spent the spring of 1900 cramming painstakingly for his diploma examinations, relying greatly on class-notes lent to him by his friend Marcel. Albert, Mileva, Marcel, Jakob Ehrat, and Louis Kollros took their final examinations. Mileva, unfortunately, did not pass.74 The exams and the struggle to cram were so exhausting and nerve-wracking that afterwards Albert lost most creative interest in physics for about a year.75 At least he had the freedom and disposition to continue some leisurely readings on his own. On rainy days in July, for example, he read “mainly Kirchhoff’s famous investigations of the motion of the rigid body,” and he commented to Mileva: “I can’t stop marveling at this great work.”76 Nonetheless, one interviewer recounted that: “almost a dislike for science and its intellectual technique remained with him after he had finished his course of study. He overcame it only a long time afterwards. He approached the broader aspects of thought through philosophical studies,
bottom of p.226


chiefly through his readings in Kant and Schopenhauer…”77 Einstein liked to read Kant and Schopenhauer partly “because they made more or less superficial and obscure statements in beautiful language about all sorts of things, statements that often aroused an emotion like beautiful music and gave rise to reveries and meditations on the world.”78
Looking Misfortune in the Face
Having earned his teaching diploma, Einstein now wanted to be an assistant at the Poly. But he was denied any position there, so he applied to various universities. He was busy applying for job after job, without any success, as he moved between various cities: Melchtal, Milan, Zurich. Away from his beloved Mileva, he visited his family and carried out minor temporary jobs. But then the two spent the winter of 1900 to 1901 together in Zurich. There, he wrote a paper on physics and submitted it to the Annalen der Physik. Mileva proudly informed one of her friends.79 He also planned to work on his doctoral dissertation.
“Once he he regained the taste, after the saturation from the knowledge crammed for his exams, for scientific work, he attacked the problem of the luminous ray which haunted him since the age of sixteen.”80 A later friend recounted: “From the time Einstein was fifteen or sixteen years old (so he has often told me) he puzzled over the question: what will happen if a man tries to catch a light ray? For years he thought about this problem.”81
Because of his travels, his conversations with Mileva were intermittent. Nonetheless, their early discussions on physics seem to have been significant.
bottom of p.227


In the spring of 1901, in a letter to her, he enthusiastically mentioned: “How happy and proud will I be, when we both together have brought our work on the relative motion victoriously to its end!”82 His creative interest in the problems of ether and relative motion had reawakened. Back then, his line of inquiry was not in kinematics but in the electromagnetic theory of light applied to moving bodies. He also had occasional discussions with friends, though having left the academic environment of the Poly. One such friend was Michele Besso. They coincided in Milan in 1900 and early 1901. Albert admired Michele as “one of the finest and most versatile talents imaginable. He was equally interested in technology, physics and sociology, and was of sound judgment in these as in all phases of life.”83 But despite Michele’s erudition, he was inefficient at his job at the Society for the Development of Electrical Enterprises in Italy. Reiterating a story told by Michele’s supervisor, Albert wrote to Mileva:
“Again, Michele has nothing to do. His manager sends him to the Casale power station to inspect and test the newly installed lines. Our hero decides to leave in the evening, to save valuable time of course, but he unfortunately misses the train. The next day he remembers his assignment too late. On the third day he gets to the train station on time, but to his horror realizes that he has forgotten what he is supposed to do. He immediately writes a card to the office saying that the instructions should be wired!! I don’t think this fellow is normal.”84
Despite such absent-mindedness, Albert relished discussing scientific questions with Michele.
In the spring of 1901, the two jointly pondered “the fundamental separation of light-ether and matter,” and “the definition of absolute rest,” among other things.85 The luminiferous ether appeared as “an incarnation” of the notion of absolute rest: “all motions of bodily objects could be referred to it, one could in that physical sense speak of ‘absolute motion’ and also found
bottom of p.228


mechanics on that notion.”86 They could discuss Mach’s comments, who critically discussed Carl Neumann’s definition of absolute rest in the hypothesis of the “body Alpha.” Such discussions were common, and other sources, soon read by Einstein, also critically discussed Neumann’s hypothetical concept.87 Einstein wanted to formulate a theory characterized by relations among observable quantities, so hypotheses about unobservable bodies seemed unsatisfactory. For most physicists an object’s rest or inertial motion could be decided only relative to an arbitrarily chosen body. Depending on where an observer stands, a given object would be seen to move or not. We don’t know what Albert and Michele said about such matters. But Albert commented to Mileva that Michele “is a weakling without any glimmers of sound human nature, who can bring himself to no achievement in life or scientific creation, but certainly has an extremely keen mind, the messy workings of which I observe with great delight. … He takes great interest in our research, even though he also often misses the big picture by the most petty scruples.”88
Nonetheless, at some point Einstein and Besso discussed the question of the relative motion of electrical conductors. Decades later Besso reminisced that it was he who highlighted the importance of electromagnetic induction: that the effect obtained from the relative motion between magnet and conductor is the same regardless of which moves, “realizing as an electro-technician that in the framework of Maxwellian theory what appears in the induced part as an electromotive force or as an electric force, depends on whether the inductor of an alternator is at rest or in rotation.”89 Visibly there was no difference in the effects. Einstein found it “intolerable” that physicists yet gave two distinct theoretical descriptions of the same effect. 90
bottom of p.229


In April of 1901 Besso and Einstein both left Milan. Besso moved, with his wife and son, to Trieste. Albert spent some days with his beloved Mileva; they were eager as usual to see each other. Yet he moved to Winterthur in search of a teaching job. By late May he knew that Mileva was pregnant.91 Meanwhile his parents bitterly opposed the impending possibility that the two would marry. Mileva also had several arguments with Professor Weber at the Polytechnic.93 This all caused great stress on her while she had to study to retake the final exams. Unfortunately, she again failed the examination. She then had more difficulties with Weber, and so she left Zurich, and decided, embittered, to not return to him.94
Despite Einstein’s efforts on the problems of relative motion, he still had “no results.”95 By September of 1901 he had conceived of yet another experiment for investigating the motion of matter relative to the ether. Einstein wrote to his friend Marcel Grossmann that the experiment would be based on ordinary effects on the interference of light, but he lacked any opportunity to carry it out.96 Einstein entertained doubts about the full relativity of motion, owing to what he soon identified as a simple mathematical error, we don’t know what, but by December 1901 he was thoroughly convinced that all inertial motion is indeed relative, even for electromagnetic processes. Albert wrote to Mileva:
“I work eagerly on an electrodynamics of moving bodies, which promises to be a capital treatise. I wrote to you that I doubted the correctness of the ideas about relative motion. My hesitations however were based on a simple calculational error. I now believe in it more than ever.”97
Two days later, he visited Alfred Kleiner, Professor of Physics at the University of Zürich, and discussed with him the questions of relative motion. Albert wrote to Mileva that Kleiner “advised me to publish
bottom of p.230


my ideas on the electromagnetic theory of light of moving bodies along with the experimental method. He found the method I’ve proposed to be the simplest and most expedient one imaginable. I was quite happy about the success.”98
In a manuscript of 1920, Einstein explained that he had been compelled to adopt the relativity of motion, through and through, because of the phenomenon of electromagnetic induction.99 Moreover, as he became increasingly aware of the failed attempts to detect the relative ether motion, it would seem pointless to carry out his own experiments on that. Indeed, “Einstein was well aware of the null results of a wide range” of such experiments.100 He recalled that “I was struck by the fact that experiments showed that the velocity of light is constant.”101 Thus Einstein came to accept, “as a fact,” the negative results of all experiments such as Michelson’s, “and I came to sense intuitively that it is our mistake to think of motion of the earth relative to the ether. … And from that time on I came to think that although the Earth goes around the Sun, its motion is undetectable by experiments with light.”102 It became just as meaningless to talk about absolute motion, independent of any reference frame, as to talk about absolute rest.
The conviction that there is no unique state of motion, which could be designated as belonging to a special frame of reference, had only gradually solidified in Einstein. Years later he explained that it had not been a “sudden” insight, and hence that by no means was it a “discovery.”103 He explained that “I was led to it by steps arising from individual laws of experience.” It had taken him several years of reflection, including thought-experiments, rough designs for plausible experiments, and some study of actual experiments to arrive at his conviction. Henceforth it became a fundamental building block for his theoretical endeavors. Years later, Einstein explained to his childhood mentor, Max Talmud, that “the empirically suggested non-existence of any such preferred ‘wind-direction’ [of ether] is the main starting point” of what eventually became his special theory of relativity. 104
bottom of p.231


Near the end of 1901, Michele Besso gave Einstein a book on the ether, published in 1885. To Einstein the book seemed hopelessly “outdated.” Instead, he resolved to study thoroughly the works of Lorentz and Paul Drude on electrodynamics, and to ask a friend from the Poly, the earnest Jakob Ehrat, to get him the available literature. Leading theoreticians, such as Lorentz, Drude, Max Abraham, and Henri Poincaré, were already extending electrodynamics much farther than the amateur outsider knew.
Thus, by early 1902, Einstein shifted the direction of his intellectual labors. In retrospect, Talmud reported that “His special relativity theory was worked out between 1902 and 1905.” 107 No longer did Einstein attempt to devise experiments to detect motion relative to the ether. He believed that the motion of all material bodies is relative to other material bodies. He began to intensively study the electrodynamics of H. A. Lorentz.
Decades later, in hindsight, Einstein noted that before 1905 he had come to know Lorentz’s booklet of 1895.108 Thereby, Einstein learned much about electrodynamics. According to Lorentz, Maxwell’s equations were only valid exactly in one reference system, distinguished from all others by its state of rest: the luminiferous ether. Lorentz analyzed electric currents as consisting of true electric masses, independent particles moving about; a conception that Einstein accepted, as he sought a description that would relate free “electricities and magnetisms” in space. He learned about Lorentz’s successful attempts to derive the Fresnel drag coefficient by way of a theory that involved the universal stationary ether. To explain the ether-drift experiments, Lorentz relied on a mathematical contrivance: the contraction of moving lengths. He posited that a body moving through the ether contracts along the direction of its motion v, by comparison to an identical body at rest in the ether, by the ratio:
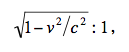
bottom of p.232


where c is the speed of light relative to the ether.109 Lorentz justified the contraction by hypothesizing that the molecular forces responsible for the cohesion of solid bodies were affected by motion through the ether, such that all bodies contract in the direction of motion, just enough to make such motion undetectable (by eliminating asymmetric path differences among light rays). Perhaps no material bodies were absolutely rigid. Thus Lorentz tried to explain the many experiments that failed to detect motion relative to the ether. Lorentz also introduced another mathematical contrivance: a term he called “local time,” which served to conveniently make the equations of electromagnetism have the same simple form (very approximately) when referred to moving bodies, as when referred to bodies at rest in the ether. Such artifices, along with the concept of the stationary ether, seemed dispensable for Einstein, who desired to establish a theory that fundamentally involved the symmetries observed in experiments. Nevertheless, Lorentz’s elegant works were immensely helpful to Einstein as to many other contemporaries.
Einstein felt that Lorentz had “completely” solved the question of the force of electrons, at least to a first approximation.110 Hence, at that time, Einstein believed that the Maxwell-Lorentz equations were definitely valid and correctly accounted for the facts.111 Yet he was unsatisfied by Lorentz’s account of electrodynamics. The elements of Lorentz’s theory did not reflect the symmetry of the phenomena, such as that of electromagnetic induction. If a wire circuit were at rest in the ether, the motion of a magnet in its vicinity was said to produce a real electric field that would set in motion the electrons in the wire. Whereas, if instead the magnet were at rest in the ether while the wire was moved, then there would be no electric field, and the same current of electrons in the wire would be said to result from the action of a hypothetical force. Einstein was unsatisfied by this “electromotive force hypothetically introduced by Lorentz.”112 He preferred to think that the symmetric electromagnetic effects of relative motion had but one underlying cause. He reached “the conviction that the electromotive force induced in a conductor moving in a magnetic field is nothing other than an electric field.”113 Einstein thus believed that “the existence of an electric field was therefore a relative one, depending upon the state of motion of the coordinate system being used.”114
bottom of p.233


Moreover, Einstein was still puzzled by Fizeau’s experiment, so he focused on theoretically examining it. By assuming the existence of the ether, along with Lorentz’s force formula, one could account for effects of moving media such as are involved in Fizeau’s experiment.115 But Einstein thought that the formula should apply with respect to an ordinary system of reference, rather than the ether. He “hypothesized” that Lorentz’s force formula would work when referred to a moving body instead of to empty space.116 One could then account for Fizeau’s results by thus using the Lorentz force and Maxwell’s equations, with “no need of the local time” concept.117 But still, the equations of electromagnetism did not exhibit complete formal symmetries when referred to systems in relative motion; they assumed complicated forms.
In early 1902 Einstein moved to the old city of Bern, Switzerland, because the father of his friend Marcel had arranged that Einstein be considered for a job there at the Federal Patent Office. By early February he found out that Mileva had given birth to a baby daughter.118 They hoped that they would soon be reunited. But rather than go to Mileva, Albert tried to secure a job. In the meantime they continued to exchange affectionate letters.
Although he arrived at beautiful Bern alone, he soon saw acquaintances. He occasionally spent time with Jakob Ehrat, from the Poly. He also spent time with Conrad Habicht, whom he had met at Schaffhausen, and who was working on a doctoral thesis in mathematics. Habicht had hobnobbed with math and philosophy people in Zurich, Munich, Berlin, and Stuttgart.119 And now he was “very enthusiastic” about Einstein’s ideas.120 Also, Einstein made a new friend: Moritz Solovine, a philosophy student at the University of Bern
bottom of p.234


who sought private lessons in physics. Solovine found in Einstein the kind of friendly teacher he had hoped for: “He presented subjects in a slow and uniform voice, but in a remarkably luminous manner. To render his abstract thoughts more easily intelligible, he sometimes took an example from ordinary experience.”121 The two got along so well that they promptly turned to discussing informally general questions in philosophy and physics.
Einstein was also visited once that Spring by Max Talmud, who found him living in considerable poverty.122 Einstein was waiting for the job at the Patent Office to materialize, while his family’s business had collapsed and his parents were unable to help him financially. Also, his father’s health was failing. And meanwhile, his fiancée and his baby daughter were still far away. Despite, such circumstances, Einstein handled himself well, as if following Schopenhauer’s advice, to acknowledge that human existence necessarily has its miseries: “If he remembers this, a man will not expect very much from life, but learn to accommodate himself to a world where all is relative and no perfect state exists—always looking misfortune in the face, and if he cannot avoid it, meeting it with courage.”123
One day Solovine and Einstein decided to jointly read and discuss works in science and philosophy. It seemed to Einstein that most scientists paid no attention to the philosophy of science, unfortunately, that it was considered a luxury.124 Solovine suggested that they begin by reading Karl Pearson’s The Grammar of Science, and so they did. In connection with Einstein’s interests, we may note that Pearson, like Mach, Hertz, Föppl, and others, emphasized relativity, asserting that one “must be careful to notice the relativity of motion; absolute motion, like absolute position, is inconceivable.”125 Furthermore, Pearson noted that
“in order to see our way more clearly through that maze of metaphysics which at present obstructs the entry into physics, we must devote some space to a discussion of the elementary notions of kinematics.”126
bottom of p.235


Einstein was already acquainted with kinematics, from his courses at the Poly, as well as his studies of Hertz and Föppl. Moreover, others sources that touched upon the subject were readily available, for example, Maxwell had written about “Electrokinematics,” in his Treatise on Electricity and Magnetism, and, Thomson and Tait discussed kinematics in their Treatise on Natural Philosophy.127 Both of these influential works were available in German translations in the 1890s. But such works did not highlight the importance of kinematics as Pearson did: as a means for eliminating conceptual obscurities.
Soon, Conrad Habicht joined Einstein and Solovine, and thus they formed a regular discussion group. The three amateurs, none of whom yet held a doctorate, decided to mockingly imitate pretentious scientific societies by giving to their group the bombastic name: “the Academy Olympia.” They held evening meetings, sometimes in one residence sometimes in another. The three would share a very simple meal, with coffee; Einstein and Habicht sometimes smoked, whereas Solovine detested tobacco in all its forms. Their lively discussions sometimes extended into the late hours of the night. Reportedly, “the conversation was often so lively and loud that the other tenants in the house did not find it a matter of spiritual enlightenment, but rather a disturbance of their night’s rest.”128
On June 23, 1902, Einstein finally began work, on a trial basis, as a patent examiner at the Swiss Federal Office of Intellectual Property. The Director, Friedrich Haller, was a strict supervisor. He emphasized the need for careful, sharp skepticism towards patent claims, along with the importance of verbal precision. Haller told the patent examiners: “When you take up an application, think that everything the inventor says is wrong. If you don’t do that, you follow the inventor’s chain of reasoning and thus you become partial. It is a matter of staying critical— vigilant.”129 Haller warned Einstein: “If you do not write clearly, I will throw you out.”130
bottom of p.236


At work, Einstein became acquainted with several other officers. One senior employee was Josef Sauter, who had also studied, years earlier, at the Polytechnic in Zurich, and had a doctorate from the University of Zurich. Sauter’s dissertation had dealt with magnetism and he had published it in the Annalen der Physik in 1897. He there also had recently published, in 1901, a paper formulating a mechanical system that accounted for Maxwell’s equations of electromagnetism. When Sauter found out that Einstein too was interested in Maxwell’s theory, he told about his own attempts to formulate a mechanical model. But Einstein replied that he was not interested in mechanical models, and remarked “I am a heretic.”131 Later, Sauter discussed with him Hertz’s work on the Principles of Mechanics, a work that aimed to elucidate mechanical foundations for all of physics. But Einstein repeated “I am a heretic.” He was interested in fundamental issues, but he did not seek an account based entirely on mechanics.
Often Solovine visited him at noon, during his lunch break, to carry on their discussions.132 On days off work, the two would sometimes meet early in the morning and converse all day as they walked around the countryside. Aside from his active interests in physics, Einstein cultivated broader interests in philosophy and literature. Einstein, Habicht and Solovine, met regularly to discuss readings in science, philosophy, and literature. As mentioned before, the first book that Einstein and company read together was Pearson’s Grammar of Science, which ascribed some importance to kinematics. Moreover, Einstein presumably became increasingly aware of the importance of kinematics from Ampère’s own writings. At some point, Einstein and his friends read together “some chapters” of Ampère’s Essay on the Philosophy of the Sciences.133 Though Einstein’s friends were not quite physicists, they could nonetheless well pursue fundamental subjects such as kinematics. Habicht, the mathematician, was interested in the subject.134 In mathematics, kinematics was often presented as the pure geometry of motion. Accordingly, some physicists, such as Hertz, and Thomson and Tait,
bottom of p.237


had presented kinematics as the a priori science of motion, a branch of pure mathematics. By contrast, Ampère’s seminal discussion argued that kinematics should be conceived as the science of motion as it appears to observation. The focus on grounding concepts on observations appealed to Einstein, being interested in the visible aspects of physics. He had puzzled over the nature of light by thinking about observers in motion.
Solovine, Einstein and Habicht also read Mach’s Analysis of Sensations, among several other works. Einstein admired Mach for his skepticism, “for his general attitude towards the foundations of physics. I see his great merit in that he had disengaged the dogmatism that reigned in the 18th and 19th centuries on the foundations of physics.”135 Einstein also admired Mach for his emphasis on problems concerning the principles of physics. Yet Einstein came to dislike the way Mach tried to connect physics and psychology, as though the objects of physical study were mere sensations, rather than things existing independently of the observer.
Meanwhile, Mileva had been lonely and anguished, living with her parents at Novi Sad.136 She had suffered from the opposition of Albert’s parents, yet she spent time in Bern, in the latter half of 1902, registering as a tenant in rooming houses.137 She did not bring their baby daughter with her. Albert and Mileva finally married on January 6, 1903, in a small ceremony witnessed by Habicht and Solovine. “That event,” Solovine recalled, “did not effect any changes in our meetings. Mileva, intelligent and reserved, listened to us attentively, but never intervened in our discussions.”138 Albert
bottom of p.238


and Mileva kept the existence of their daughter a secret. Was she given up for adoption? Some friends of Mileva noticed a change in her attitude, she seemed to brood about a problem; they inquired but she insisted that it was just too personal, “intensely personal.”139 Nevertheless, Albert and Mileva were glad to be together.
Now that Einstein had some peace of mind, he dedicated time to his theoretical investigations. His job at the patent office consumed many hours of each day. It even required him to attend training sessions on Saturdays. Moreover, the majority of the patents that he had to evaluate, appeared as “indescribably trivial and lifeless.”140 Nevertheless,
“He soon discovered that he could find time to devote to his own scientific studies if he did his work in less time. But discretion was necessary, for though authorities may find slow work satisfactory, the saving of time for personal pursuits is officially forbidden. Worried, Einstein saw to it that the small sheets of paper in which he wrote and figured vanished into his desk-drawer as soon as he heard footsteps approaching behind his door. If he had been discovered, he would have been ridiculed as well as harmed. The Director would have laughed at him in addition to being angry…” 141
The discretion seems to have worked. One colleague, Dr. Josef Sauter, got the impression that Einstein’s daily duties were followed by nightly investigations in physics.142 In one place or another, he managed to continue his projects. He worked on several subjects, including the foundations of thermodynamics, on which he published a few papers in the Annalen der Physik. And he discussed such published papers with Sauter and others. But above all, Einstein continued to be concerned with the problems of electrodynamics. Reportedly, he explained to his wife his conviction about the relativity of motion as follows: “If I am riding on a train,” he said to Mileva, “a raven flying at the exact speed of the train will seem to be making no progress. But a person standing on the ground will say that the raven is flying fast. All motion is relative because it is measured differently by different observers.”143 Examples of this kind
bottom of p.239


were quite traditional and easy to understand. Yet his main question was “How can one extend the relativity to the electro-magnetic field?” 144
Ruminations of the Hypothetical Sort
Einstein struggled to formulate a simple intuitive theory based on the symmetries of relative motion. To reproduce Lorentz’s successful results without using the ether concept, Einstein presumably would have to account for known experimental effects without using the artificial mathematical devices of length contraction and local time. Why would a rigid body contract as it moves against the ether, if no such ether could even be detected? Moreover, why would all bodies contract by the same length though they consist of various substances? Algebraically, length contraction served to make Lorentz’s theory yield the known experimental results. But physically, this hypothesis seemed artificial. Einstein recollected:
“This hypothesis formally sufficed for the facts of the situation, but in spite of that the mind remained dissatisfied. Should nature have placed us really in an ether gale, and on the other hand exactly so arranged the laws of nature that we can notice nothing of this gale?” 145
For Einstein, as for other physicists, the hypothesis of length contraction, “This assumption introduced ad hoc, seemed yet but only an artificial expedient, for saving the theory” of Lorentz, for making it agree with experimental results.146 Such artifices seemed ultimately dispensable.
Einstein knew that not only hypotheses, but also the so-called “laws” of physics might be susceptible to revision. Reportedly, his early readings of Kant had influenced his outlook:
“Kant had decided that man-found laws of science which stood up to test derived from the inventive element of human reason as well as from experience. Einstein chewed on this for a long time. If the human mind could invent as well as reason logically, then intuition and imagination were the keys to creation. They could play tricks, but they could also lead to the discovery of a truth that had not been known before.” 147
bottom of p.240


To contribute to theoretical physics Einstein turned to the inventive imagination. He had to advance his own hypotheses. This process could be guided by intuition, by past experiences.
Again, Einstein pondered the mysterious nature of light. If maybe the ether did not exist, then light would not consist of waves in a medium. Presumably, light would consist of a fine granular material, tiny particles spreading out freely across empty space. Since 1899 he already entertained the idea that the agents of electromagnetic actions might move freely in space. Corpuscular conceptions of light had been espoused in the distant past by numerous physicists. For one, he knew that Newton had espoused a corpuscular theory of light, although he did not study Newton’s writings in depth.148 According to classical mechanics, and plain experience, the velocity of a projectile depends on the velocity of its source at the instant of emission. Therefore, if light were a projectile, rather than a wave, its propagation should depend on the motion of its source at the instant of emission. In mechanics, the relativity of motion was assured precisely because the behavior of all bodies projected within an inertial system would all be affected equally by its state of motion, such that there is no net difference among their motions. Accordingly, if perhaps light behaved like a projectile then no experiments involving stationary light-sources would reveal any motion of the experimental system. As the ether-drift experiments had all failed, Einstein was reasonably justified in expecting that light obeyed the relativity of motion. So he adopted the hypothesis that the speed of light depends on that of its source.149
Relying on this hypothesis, Einstein tried to devise a theory of electrodynamics. Physically, the hypothesis was compatible with experiments in electrodynamics and optics. For example, it agreed with the phenomena of the aberration of starlight in telescopes. This effect can be interpreted according to the relativity of motion together with the hypothetical source-dependence of the speed of light: as the star moves relative to the Earth, the starlight is projected with a velocity that depends on that of the star. The relative
bottom of p.241


velocity of starlight seemed to consist of a simple vectorial sum. Moreover, perhaps Einstein’s hypothesis would serve to explain Fizeau’s result (and thus the Fresnel coefficient) by somehow accounting for the relative motion between the moving water and the stationary source of light.150
However, the hypothesis seemed to entail some odd novelties. One example was that a train of light signals could reverse its sequence: if light were emitted from an accelerating source then the signals emitted first would move slower than those emitted afterwards, so that eventually the latter would overtake the former.151 This theoretical effect seemed unnatural to Einstein, as no such phenomena was known. Still, the theoretical possibilities were considerable, and his hypothesis seemed promising.
Meanwhile, the meetings of the Academy Olympia continued. For Einstein these meetings were great fun, as they “delighted with a childish joy in all that which was clear and reasonable.”152 According to Solovine, “the foundations and the principles of the sciences” were their constant preoccupation.153 He also noted that “In the examination of fundamental notions, Einstein employed with predilection the genetic method.” That is, that to “clarify” fundamental notions, Einstein appealed to “that which he had been able to observe in children.”154 At a young age, he intensely studied a geometry book that mentioned the “genetic clarification” of a concept, of “specifying in which way it can develop.”155 This sort of expression in formal treatises followed Kant’s use of “genetic definition” in his Logic of 1800.156 Yet the “genetic method” identified by Solovine in Einstein, referred to the development of ideas in children, so it was more in the realm of developmental psychology.
bottom of p.242


At the time, the genetic method aimed to investigate the formation of ideas and habits, to replace static metaphysical presuppositions with scientific statements. Owing to Darwin’s explanation of the variations of species, notions of evolution were being increasingly applied to the sciences. In the 1890s, James Mark Baldwin pioneered this approach in psychology by performing systematic experiments on children, advocating “the genetic method.” Einstein actually read a few texts by authors who voiced interest in the growth of concepts in children. In his Analysis of Sensations, Mach argued that “the aim of all scientific research” is the “adaptation of thoughts to facts” and that that process can be essentially observed in children.157 Mach sought to understand the gradual development of abstract concepts and intuitions; and for that, he pondered how people develop such notions, especially at early ages. He even gave examples from what he had been able to observe in his own child. Furthermore, Hermann von Helmholtz argued that a person’s knowledge of axioms of geometry and principles of mechanics stems not from transcendental sources, but from ordinary everyday experiences.158 Throughout the years, Einstein maintained a playful interest in how children learn.159
On the evening of his twenty-fourth birthday, on 14 May 1903, Einstein lectured to his friends at length about “the Galilean law of inertia.” As usual it seemed that “when he boarded a problem he would forget completely the Earth and its miseries and joys.”160
bottom of p.243


That day he was so absorbed in his account of inertia that he entirely ate, without even noticing what it was, all the expensive caviar that his friends had bought for him as a gift. That same year, as a gesture of appreciation, Solovine presented Einstein with a humorously flattering “Dedication,” as “Member of the Academy Olympia.” It included a drawing of Einstein’s head as a sculpture, and it read:
EXPERT IN THE NOBLE ARTS. VERSED IN ALL LITERARY FORMS – LEADING THE AGE TOWARD LEARNING, A MAN PERFECTLY AND CLEARLY ERUDITE, IMBUED WITH EXQUISITE, SUBTLE, AND ELEGANT KNOWLEDGE, STEEPED IN THE REVOLUTIONARY SCIENCE OF THE COSMOS, BURSTING WITH KNOWLEDGE OF NATURAL THINGS, A MAN OF THE GREATEST PEACE OF MIND AND MARVELOUS FAMILY VIRTUE, NEVER SHRINKING FROM CIVIC DUTIES, THE MOST POWERFUL GUIDE TO THOSE FABULOUS, RECEPTIVE MOLECULES, INFALLIBLE HIGH PRIEST OF THE CHURCH OF THE POOR IN SPIRIT. 161
Above the picture of Einstein’s bust, Solovine drew a hanging line of sausage links.
One day, at a bookstore, Habicht noticed and bought a recent publication that seemed promising: Science and Hypothesis, by the mathematician Henri Poincaré; first published in 1902.162 Habicht and company read this book at their Academy, and according to Solovine, it “engrossed us and held us spellbound for weeks.”163 Poincaré lucidly explained the important functions of hypotheses in mathematical and experimental physics. He even discussed the functions of hypotheses in mathematics.
Traditionally, most mathematicians had believed that the axioms of geometry were necessary and self-evident truths. But following the invention of non-Euclidean geometries, increasingly more mathematicians admitted that some of the axioms of Euclid’s geometry were based on physical hypotheses, generalizations from experience. This outlook had been fostered thanks to the work of Bernhard Riemann, “About the Hypotheses that Lay at the Foundations of Geometry,” which was also read
bottom of p.244


by Habicht, Einstein, and Solovine. Poincaré reviewed such developments and argued that the axioms of Euclidean geometry are “conventions” freely chosen in accord with ordinary empirical knowledge.164 He argued that Kant had been wrong in construing the axioms of geometry as a priori judgments. Different axioms could be adopted, as in the geometries of Riemann and others. Furthermore, Poincaré explained that some definitions in traditional geometry were accepted merely as assumptions. For example, the definition that figures are equal if they can be superposed exactly, presupposes the axiom that a rigid body can be moved without altering its shape when it returns to rest. But to know whether a figure changes when moved we would need a way to establish the equality of figures. Thus Poincaré noted that this definition involves a vicious circle, and the usual ideas about the motion of rigid figures were not self-evident truths.165
Science and Hypothesis was also the only work among those read jointly by Solovine, Einstein and Habicht that discussed electrodynamics and Lorentz’s theory. After analyzing fundamental mathematical and empirical conditions under which physicists labor, Poincaré illustrated the development of hypotheses in the history of optics and electromagnetism. He reviewed the ideas of Fresnel, Ampère, Maxwell, Helmholtz, Lorentz, and others. Above all, Poincaré argued that, to date, Lorentz had formulated the most satisfactory and serviceable theory of electrodynamics. He praised Lorentz’s theory for its simplicity in accounting for the optics of moving bodies, especially for Fizeau’s experiment.166 But he complained that Lorentz’s seductive theory violated Newton’s law of action and reaction, inasmuch as electrons could allegedly act on the ether without there being any immediate and observable reaction. Moreover, Lorentz’s mathematical theory was not rigorously compatible with the principle of relativity. Poincaré disliked that Lorentz had introduced convenient constructs precisely whenever experiments did not agree with theoretical predictions; he preferred that a theory should rigorously agree with phenomena from within, rather than by the addition of auxiliary fixtures.
bottom of p.245


Poincaré dedicated an entire chapter to discussing questions about relative and absolute motion. He placed great importance on “the principle of relative motion.” The “contrary hypothesis,” that phenomena, when referred to systems in inertial motion rather than stationary, might be described by different laws, seemed “singularly repugnant to the mind.”167 He expected that all experiments ever would disclose only relative motions among material bodies.168 He alluded to the history of old hypothetical subtle fluids, of heat, of electricity, which were eventually abandoned, and accordingly he asked: “And does our ether really exist?” (In 1888 he had anticipated that “no doubt, some day the ether will be thrown aside as useless.”)169 Yet he admitted the ether as still a very convenient hypothesis. He concluded Science and Hypothesis by noting that even if some experiment were to succeed in detecting what seemed to be absolute motion, that still, for the followers of Lorentz, “the principle of relativity is safe” because any such seemingly absolute velocity would be understood as really just a velocity relative to the ether (rather than to absolute space).170 Thus one of the benefits of the ether hypothesis was that it served to safeguard the principle of relativity.
Aside from acknowledging that the ether hypothesis could be preserved, Poincaré thus had asserted some of the ideas that Einstein now entertained. Some of the other ideas Einstein entertained had likewise been voiced by a few of the writers with whose writings he was familiar. For example, Wilhelm Ostwald had suggested that the ether hypothesis could well be rejected, in a work Einstein read.171 Likewise, John Stuart Mill, while discussing “the Hypothetical Method,” gave skeptical reasons against “the prevailing hypothesis of a luminiferous ether,” in his System of Logic, a work Einstein read with Solovine and Habicht.172 Furthermore, Mill remarked on several phenomena that seemed to be more amenable to explanation on the hypothesis that light propagates as projected corpuscles.173
bottom of p.246


Though Einstein entertained appealing concepts, he still failed to find a solution to the problems of relative motion in electromagnetic theory. In particular, his hypothesis about light seemed to involve theoretical and mathematical difficulties. One difficulty was exactly how to explain Fizeau’s experiment, and Einstein found no straightforward way to do so on the assumption that the speed of light depends on that of its source.174 Again, he could not escape the old conflict between the negative results of the ether-drift experiments, and the apparent slight dragging of light in Fizeau’s experiment: “This contradiction remains, even if we assume a different hypothesis, not involving the ether, for Fizeau’s experiment.”175 Another difficulty was how to explain the formation of a shadow by a screen moving relative to the light source.176 Einstein conjectured that one would have to introduce “the ugly assumption” that the kind of light emitted from a resonating source depends on the velocity of the light that first impinges on it.177 Also, he expected that light approaching a mirror perpendicularly at a speed of c + v would have to be reflected at a speed of c – v instead of keeping its same speed.178 The ensuing mathematical difficulties seemed formidable. He therefore increasingly disliked the idea of characterizing light by various speeds, it seemed easier to follow tradition by defining light only by frequency and intensity.179 Einstein also surmised that the speed of light passing through a film could change in such a way that interference effects “would give rise to entirely unbelievable phenomena.”180 Furthermore, he apparently imagined that light of different colors would travel at different speeds; but it then “seemed absurd” that yet all the light emitted from any stationary body, of whatever color, has the same speed.181 To him this became the “strongest argument,” a priori, against the
bottom of p.247


hypothesis that the speed of light depends on that of its source. This argument also had an empirical component, at least within the range of observational precision available. Namely that, if light of different colors propagates at different speeds, one would expect that when a star is eclipsed and then uncovered, then for the first moment after it is visible, starlight of all colors would not reach us simultaneously.182 But no such effect was known.
Still, such arguments were mainly theoretical, not experimental, so Einstein struggled to develop this imaginable way of establishing full relative motion. It seemed that if he were to succeed, then the theory of the optics of moving bodies would become increasingly complicated. But even if such difficulties could be overcome, there remained a greater difficulty. Einstein did not find any way to formulate a mathematical theory of electromagnetism compatible with his hypothesis. He sought differential equations that would allow that in every direction electromagnetic waves may propagate with different speeds. But finally, “He gave up this approach because he could think of no form of differential equation which could have solutions representing waves whose velocity depended on the motion of the source.”183 Having reached this mathematical impasse, Einstein turned to look for another “way out.”184
Returning to Lorentz’s theory, Einstein weighed what seemed more plausible: that the speed of light depends on its source or that it does not.185 Lorentz’s theory accounted very well for the optics of moving bodies, whereas Einstein’s alternative scheme entailed complications. He therefore decided to abandon his hypothesis and instead adopt again the common “hypothesis of the independence of the velocity of light” from the motion of its source, owing to “its simplicity and easy practicability.”186 Even though Einstein conceived of radiation as free structures in empty space, he could not
bottom of p.248


ascribe to them the common-sense notion that their speed depends on the state of the source at the moment of emission. So now he supposed that even if light were made of particles, they would behave like waves in the sense that all light signals would have the same speed. This was a counterintuitive assumption. Einstein had begun to accept that “a theory fundamentally different from that of Lorentz, which would be based on simple and intuitive assumptions and would accomplish the same ends, could not be formulated.”187 He still rejected the stationary ether, but he borrowed Lorentz’s fundamental hypothesis that “every light ray in a vacuum always propagates (at least with respect to a certain coordinate system K ) with the same definite constant velocity.”188
The constancy of the speed of light was a physical hypothesis and a convenient way of organizing empirical data. Again, Einstein appreciated the function of concepts in physics; some common concepts were posited by the imagination for the purpose of systematically organizing and analyzing experiences. But some concepts could turn out to be unnecessary, if only there were a more economical way of accounting for phenomena. Einstein surmised, in particular, that the concept of motion relative to an undetectable ether was superfluous. It had even engendered additional concepts that seemed artificial, such as length contraction. Lorentz had tried to justify the latter with hypotheses about intermolecular forces. The concept of “local time” also seemed artificial, lacking explanation. Nevertheless, such concepts exerted a suggestive power.
The concept of local time was significant because it simplified the equations of electrodynamics, and further: because it constituted a rare example of innovation in the notion of physical time. Poincaré came to construe it as the “most ingenious” idea in Lorentz’s electrodynamics.189 Guided by
bottom of p.249


the results of experiments, Lorentz had contrived a useful mathematical construct, an artificial kind of time. Here indeed was a “concept.”190 Traditionally, several philosophers believed that true time was not a concept in this sense. For Kant, especially, time was not a “concept,” and could not be made to depend on others. He claimed that the “axioms” or “principles” of time “cannot be drawn from experience, for this would yield neither strict universality nor apodictic certainty.”191 Time was known intuitively as an a priori truth underlying the very possibility of all perceptions. Kant argued emphatically that, “Time is not an empirical concept,” it is not derived from experience. Schopenhauer had also asserted similar claims.192 Einstein was familiar with such ideas. From age thirteen he had pored over Kant’s Critique of Pure Reason. At seventeen, while his friends often attended parties to drink beer, he would instead get “intoxicated on Kant’s Critique,” saying that “beer makes one dumb and lazy.”193 And, he had further studied Kant’s philosophy at the Zurich Polytechnikum in 1897, in a course that included a discussion of Kant’s ideas on space and time.194 Philosophers and metaphysicians thus analyzed “true time.” By contrast, Lorentz’s local time was a concept that, precisely, claimed no universality or certainty.
In Bern, studying fundamental issues in philosophy and science, Einstein, Solovine and Habicht read several works that critically discussed notions of time. They discussed Mach’s book on mechanics (which Einstein had earlier read), where Mach denounced the notion of absolute time as an idle metaphysical fiction. Likewise, Pearson, in his Grammar of Science, had argued that “time is a relative order of sense-impressions … there is no such thing as absolute time,” and, that one must not “project the absolute time of conception into a reality of perception.”195
bottom of p.250


Baruch Spinoza (a work for which Einstein felt great affinity subsequently) where Spinoza attempted to prove, among many other things, the proposition that, “We can have only a very inadequate knowledge of the duration of things external to us.”196 Moreover, they discussed Science and Hypothesis, the book that “engrossed us and held us spellbound for weeks.”197 There Poincaré claimed that:
“There is no absolute time; to say two durations are equal is an assertion that by itself has no meaning and which can only acquire a meaning by convention. Not only have we no direct intuition of the equality of two durations, but we have not even that of the simultaneity of two events produced in different theaters; this is what I have explained in an article titled ‘The Measure of Time.’”198
Among the other works they jointly read, a few touched upon the narrow concept of simultaneity in relation to other aspects of time. In his System of Logic, John Stuart Mill discussed the character of propositions concerning “Order in Time” in terms of the notions of “Sequence” (succession) and “Co-existence”
(simultaneity).199 In his “Numbering and Measuring,” Hermann von Helmholtz discussed in passing the question of “Time measurement,” stressing that the equality of durations is ascertained on the basis of whether or not physical processes begin and end “simultaneously.”200 Also, in his Grammar of Science, Karl Pearson argued that a “mode of discrimination” of Abstract Science must concern the analysis of sequence in Time, because “the immediate groups of sense-impressions are not really simultaneous, and most things perceived in space are ‘constructs’ involving stored sense-impresses.”201
bottom of p.251


Moreover, Einstein, in particular, was in a position to be especially aware of the neglected but significant notion of simultaneity, owing to his early interest in Kant, who had analyzed it at length.202
In 1770, Kant criticized Leibniz and other philosophers for their view which “at once shows itself erroneous by involving a vicious circle in the definition of time, and also by entirely neglecting simultaneity, a most important consequence of time.”203 Kant also discussed the concept of simultaneity in his work of 1781, Critique of Pure Reason. From Kant, Einstein could learn to subdivide the notion of time into the notions of duration, succession, and simultaneity, and to appreciate the fundamental role these notions play in human perception.204 Kant denied the idea that time has any absolute reality, at least in the sense that time could not be said to be “objectively real” if abstracted from the sensibility of intuition and applied to “things in general.”205 Time only had “objective validity” in respect of appearances.206 Moreover, Kant argued that the temporal ordering of events in space must be constructed according to ideas about physical interactions.207 Again, time was not a concept, it could not depend on experience. Kant argued that “the proposition that different times cannot be simultaneous cannot be derived from a general concept.”208 Einstein could become further acquainted with such ideas through the works of Schopenhauer. Following Kant, Schopenhauer denied the absolute existence of time, emphasized its
bottom of p.252


subjective origin, and made significant use of the notion of simultaneity.209 Still, Schopenhauer also commented on variations in our perceptions of time. In his Parerga and Paralipomena, which Einstein read, Schopenhauer noted that a month seems longer when people travel than when they stay at home, and also, that time seems to go faster as people get older.210 He also commented on the problem of being right when everyone else is wrong, as follows:
“He who can see truly in the midst of general infatuation is like a man whose watch keeps good time, when all clocks in the town in which he lives are wrong. He alone knows the right time; but what use is it to him? for everyone goes by the clocks which speak false, not even excepting those who know that his watch is the only one that is right.”211
We do not know whether any such varied statements provoked any interest in Einstein, but at least such were the comments about time which were voiced by some of the authors he read.
Furthermore, Einstein, Habicht and Solovine could have pursued in full Poincaré’s discussion of the measurement of duration and simultaneity by seeking his article of 1898. But even if they didn’t obtain the original publication, it was reissued in Poincaré’s latest anthology, The Value of Science, published in 1905, which they read sometime that year.212 Moreover, and earlier, if Einstein or either of his friends read Science and Hypothesis in its German translation of 1904, they could read an editorial note on Poincaré’s arguments on simultaneity, including a translation of the relevant passage from “The Measure of Time.”213
In his article Poincaré analyzed fundamental problems about the measurement of time. He asserted the need for specifying the exact procedures used to determine the duration of any physical process, as well as the simultaneity of events. He stressed that “we have not a direct intuition of simultaneity, nor of the equality of two durations.”
bottom of p.253


Decades earlier, a few theorists, such as Siméon Denis Poisson, Auguste Calinon, and Ludwig Lange, had noted that there exists a logical circularity between the concepts of time and velocity. Following Calinon, Poincaré emphasized the logical circularity in any definition of velocity in terms of time, and vice versa. The essential difficulty is that to measure the speed of a signal traveling in a single direction, one needs to know the instants when the signal passes the endpoints of a given distance, and these times must be obtained from synchronized clocks. The measurement of any one-way speed presupposes knowing the simultaneity of distant events. But the synchrony of clocks at distant places can be set or verified only by means of signals of known speed. Years later Einstein noted that “It would thus appear as though we were moving in a logical circle.”214
Poincaré argued that the only way to then establish velocities and simultaneity was by means of some convention. He explained:
“When an astronomer tells me that some stellar phenomenon … happened [at a certain time] … I seek his meaning, and to that end I shall ask him first how he knows it, that is, how he has measured the velocity of light.
“He has begun by supposing that light has a constant velocity, and in particular that its velocity is the same in all directions. That is a postulate without which no measurement of this velocity could be attempted. This postulate could never be verified directly by experiment...” 215
Terrestrial and astronomical measurements presuppose the equality of the speed of light in different directions. This was a useful convention. Poincaré noted that the assumed constancy of the speed of light “furnishes us with a new rule for the investigation of simultaneity.” One could call distant events “simultaneous,” by convention, by coordinating distant clocks using light signals assumed to travel at the same speed.
Poincaré’s concern with practical measures of time found a useful theoretical application in electrodynamics. He soon realized that Lorentz’s concept
bottom of p.254


of “local time” could be explained in terms of the synchronization of clocks. In a paper from December 1900, Poincaré pointed out that the fictitious times that Lorentz had ascribed to moving reference systems, that is:
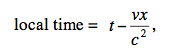
where t is the true time, would actually be obtained by observers moving together but separated by a distance x, who synchronized their watches by exchanging light signals.216 The procedure can be illustrated as follows.217 Suppose that two observers stand on opposite ends of a platform moving at a velocity v with respect to the ether. One sends a flash of light to the other, across the length of the platform x. Since the speed of light is taken to be independent of the speed of its source, the signal travels at speed c – v relative to the platform, so that it takes a time x/(c - v) to reach the “receding” observer. In turn, that observer sends a signal back, which travels at a relative speed of c + v, so that it reaches the first observer after a delay of x/(c + v). The total lapse for the light signal to go from one clock to the other and back is just the sum of the two:
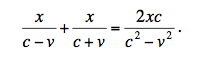
Not knowing their motion relative to the ether, the observers would assume that the light takes half of the total lapse to go from a clock at one end of the platform to another at the opposite end. The discrepancy between that inference and the real travel time would be:

bottom of p.255


Since a moving clock would not be synchronized with a clock at rest in the ether, the time of any event given as t for the stationary clock would instead be:
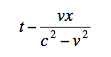
for the moving clock. The square of the speed of light was known to be far, far greater than, say, the orbital velocity of the Earth, thus one might well disregard the term v2. Hence Poincaré could identify Lorentz’s local time with the apparent time of moving clocks thus synchronized.218 Besides, Lorentz’s algebraic expression for the local time was just an approximate value. (Moreover, an additional correction could be made by taking into account the contraction of moving lengths, but the approximate apparent time remains the same.) The important point is that Poincaré found a physical meaning for Lorentz’s seemingly arbitrary concept.
In his 1900 paper, Poincaré formulated his argument succinctly, in words, but any physicist could easily carry out a calculation like the one above. If Einstein read such lines he would readily grasp that Lorentz’s local time could be construed in terms of plausible physical procedures. Poincaré’s article of 1900 appeared in a celebratory volume dedicated to Lorentz, which, virtually, “was read by every expert in the field” of electrodynamics.219 (By early 1906, at the latest, Einstein was familiar with it, because in a paper written in May he cited Poincaré’s paper.)220 Moreover, Einstein and his friends could later encounter the same argument in yet another one of Poincaré’s papers in his popular anthology of 1905.221 There too Poincaré explained that moving observers can adjust their clocks with light signals such that they obtain Lorentz’s local time. But he noted “It matters little, since we have no way of perceiving it.”222
bottom of p.256


Poincaré, complained that in Lorentz’s theory, even the novel concept of local time was not enough for a systematic accounting of phenomena, but that “complementary hypotheses” were also needed. In particular, he complained that physicists had to hypothesize the existence of length contraction and of forces that precisely compensate the effects of inertial motion on gravity, elasticity, and electromagnetism, all to explain why motion relative to the ether could not be detected. This accumulation of artificial hypotheses suggested that Lorentz’s theory was not final, but that aspects of it would have to be changed. In Einstein’s words: “This sort of way of accommodating experiments with negative outcomes through ad hoc contrived theoretical hypotheses is very unsatisfactory.”224
In July 1903, Habicht had completed his dissertation in mathematics at the University of Bern. Afterwards, he moved back to Schaffhausen, where his family lived. Einstein was annoyed to have their Academy strained by distance, so he often sent letters to Habicht, trying to encourage his visits. He denounced him as a “miserable sloth.”225 Habicht’s occasional visits were not enough: “Do come again, you miserable creature, you most pitiable of all the membra academica who ever inhabited this world! I would scold you even more, but space doesn’t allow it.”226 In the meantime, Einstein attended some meetings of the Society of Natural Inquiry in Bern. He gave a talk on December 5, titled “Theory of Electromagnetic Waves.”227
In early March 1904, Solovine too left Bern, moving to Strasbourg, and soon to Lyon, to visit the local university. So Einstein was separated from his main conversation partners, aside from Mileva, who was then pregnant.
bottom of p.257


Apparently, scientific discussions with her were lacking. A later friend and colleague of Einstein recounted that “When he wanted to tell her, as a fellow specialist, his ideas, which overflowed from him, her reaction was so scant and faint, that often he just did not know whether she was interested or not.”228 Still, Einstein continued his correspondence with friends. His willingness to simplify physical theories is illustrated in a letter to Marcel Grossmann: “You treat geometry without the parallel axiom, and I treat the atomistic theory of heat without the kinetic hypothesis.”229
On May 14, 1904, Mileva gave birth to a son, whom they named Hans Albert. Meanwhile, Einstein had earlier encouraged his good friend Besso to apply for a job at the Patent Office. Besso had been working in Trieste, as a freelance engineer, but was willing to take up a more regular employment. Luckily for both of them, Besso was granted the job as a patent examiner and began work in the Summer of 1904. Living now in the same city, Einstein and Besso renewed their discussions. After work, the two would often converse about many subjects, including physics, mathematics, and philosophy.230 Twenty years later, Einstein expressed his appreciation for Besso’s “brilliant capabilities and his extraordinary knowledge in technological and purely scientific matters.”231 He was also a good listener. Reportedly, “He was the only one possessed of enough patience to listen long hours to Albert.”232
At the Federal Office, examiners had to carefully inspect applications to judge their merits and claims to novelty. The description of every new device had to be compared to other devices listed in patent records. The proposals submitted for examination were very diverse, especially owing to the rapid growth of electrical technologies. There were proposals for lamps, motors, engine components, batteries, and many other devices.233
bottom of p.258


Many contrived gadgets seemed worthless, but on occasion, some appealed to Einstein. Years later he recalled having liked the design for “an improved slide rule.”234 Sometimes, during slack moments at the office, Einstein and Besso discussed “sensible subjects,” such as physics.235 On some days, after work,
“they went to a café patronized by university professors and assistants for talk and coffee. It was always coffee because Einstein did not drink wine and he regarded beer as poison. Tobacco, on the other hand, was part of his diet. He was addicted to cigars—the longest, fattest, blackest ones he could find. He smoked one after the other so that, even as a young man, his teeth were stained brown and his throat was raw.” 236
Einstein sometimes met applicants for patents, such as Julius Friedrich Ries, a graduate student of physiology who designed a blood lancet. Ries reported the following chat from 1904:
“This example came to me as a kind of thought-flash while reading [Ostwald’s] ‘Naturphilosophie.’ I explained the matter to Einstein in the following way: Suppose that I am sitting in a railway carriage and it travels at the speed of light along a wall of wooden boards. I have a big pot of paint and a paintbrush. With these I can then make a brushstroke on the wall at particular intervals of time, e.g., every second. Thus I would divide the wall into sections of 300,000 km each. Now suppose also that the train travels from infinity to infinity. But then it always stays ‘equally’ distant from both endpoints; so, according to mathematical theory, the train does not move from its location, so here the concepts of space and time must collapse. – I was astonished by how seriously Einstein took this example and how often he returned to it.” 237
bottom of p.259


Back home, Albert and Mileva occasionally invited friends, such as Michele and his wife Anna, for an evening at their apartment. To entertain, Albert sometimes played the violin. Often Mileva “would start the guests off on a subject that she knew interested Albert and then sit back and listen. He argued with gusto and laughed uproariously at the least excuse.”238 Still, Einstein missed the “academic” meetings with Habicht and Solovine.
Despair on the Path to Insanity
Meanwhile, Einstein continued to struggle with electrodynamics. The problem of relative motion “was always with him.”239 He still had not solved it. To some extent, however, “the arguments and building blocks were being prepared over a period of years.”240 Reportedly, “His method was to work from basic truths and try every conceivable deduction, no matter how ridiculous it seemed.”241 He later characterized his labors as “desperate efforts.”242
Like Poincaré, he now believed that Lorentz’s theory included many valuable contributions, but that some parts of it needed to be replaced or revised. Hence he began to try to somehow modify Lorentz’s electrodynamics.243 The concept of the ether, he thought, hindered the possibility of symmetrically describing electromagnetic effects referred to bodies in relative motion. He disliked that the ether concept had entailed various other unobservable hypotheses, such as the electromotive force, the contraction of rigid bodies, and the concept of local time. He expected that the equations of electromagnetism should apply in their basic form when referred to bodies in motion. The laws of electromagnetism, like the laws of mechanics, should have the same form when applied to bodies “at rest” as when applied to bodies in uniform rectilinear motion. The example of electromagnetic induction had convinced him that the relativity of motion had to be rigorously valid in electrodynamics. Various experiments in the optics of moving bodies seemed to confirm this conjecture. He believed that fundamental physics should describe relations among empirically meaningful concepts, rather than, say, relations among unobservable metaphysical structures, such as “absolute” space and motion.
bottom of p.260


He had adopted the widespread hypothesis that the speed of light is independent of the motion of its source at the instant of emission. This hypothesis was at the heart of Lorentz’s electrodynamics; why would all light move at the same speed if not because it all consisted of waves in a medium? But Einstein wanted to formulate a theory independently of any such medium. According to one writer, Einstein and Besso, allegedly, exchanged the following words:
“Do you suppose ether simply does not exist?” he asked Besso. “Newton proved its existence,” Besso protested. “Did he?” asked Einstein. “Couldn’t it have been just a fabrication to account for something for which he and other scientists had no explanation?” Besso chuckled. “Sometimes I wonder about you, Albert.” He tapped his head significantly. Einstein grinned and dropped the subject, but in his own mind he had already abandoned the concept of ether. 244
Light was very well known to exhibit effects that were understood only in terms of the idea that light consists of transverse waves in a medium. The propagation of light from the stars to the Earth suggested that outer space, the apparent voids between the heavenly bodies, were not empty. Also, Newton had considered the existence of an ether at least in order to explain the transmission of forces across the spaces between heavenly bodies.245 Even Mach was willing to admit the existence of the electromagnetic ether. By contrast, Besso’s younger friend disliked this ether concept, though he lacked alternative explanations for key optical phenomena.
Einstein was not the only physicist trying to reformulate electrodynamics without this ether concept. In particular, the well-known theorist Emil Cohn labored to revise the Maxwell-Lorentz equations to describe electromagnetic processes only with respect to observable bodies.246 Following Mach, Cohn avoided speculations about micro-physical structures and processes. He even avoided the concept of empty space. He
bottom of p.261


rejected Lorentz’s hypothesis of length contraction since it involved arbitrary assumptions about molecular forces. Following Poincaré, he interpreted Lorentz’s local time as the time given by clocks synchronized by light signals on a moving system. He argued that Lorentz’s theory required ideal measuring rods and clocks in order to distinguish Lorentz’s distorted quantities from true coordinate relations. Cohn required that light in any homogenous medium would propagate at the same speed in all directions. His theory also involved the tacit assumption that the speed of light depends on the speed of its source. Cohn did not believe that electromagnetic theory should be reduced to mechanical principles, such as the principle of relative motions. Instead, he allowed that a kind of absolute motion could be defined by referring velocities of bodies (like the Earth) to the stars.
Another physicist who already had rejected the ether concept was Alfred Bucherer. He argued that only relative motions among material bodies could produce variations in observable phenomena of light, electricity and magnetism. In 1903 Paul Nordmeyer, under Bucherer’s direction, had demonstrated experimentally that the intensity of light rays remained constant irrespective of their directions. Bucherer denounced the concept of the stationary ether as an artificial “scaffolding” that had not led to observed effects and should therefore be eliminated.247 He further rejected Lorentz’s hypothesis of contraction as very artificial. Bucherer argued that Lorentz’s equations had to be somehow reinterpreted such that all velocities involved would be only relative. (At any rate, we do not know whether Albert Einstein, for one, was acquainted with works such as those of Cohn and Bucherer in 1904.)248
But even without an ether, the problems of electrodynamics did not seem easy to solve. In any case, the speed of light should still be susceptible to apparent variations. If there were no ether, then light could only move at a constant velocity relative to something else, such as relative to space itself. But here one returns to the unverifiable concept of absolute space. Otherwise, light might have a constant velocity relative to a given frame of reference, such as a material medium, a given body, or the stars. But then light rays traveling
bottom of p.262


with the same speed in opposite directions relative to that one system could yet have different velocities when relative to other systems. Moreover, even if light were independent of the motion of its source, its relative speed should still depend on the motion of reference systems. For example, a single ray of light observed from two reference systems moving in opposite directions could seem to travel with different speeds relative to each system.
The optical experiments that continued to be most influential to Einstein were the well-known effects of the aberration of starlight in telescopes, and Fizeau’s results on the speed of light in moving water.249 Einstein’s investigations continued to be guided by “the desire to make physical theory fit observed fact as well as possible.”250 He believed that the equations of electromagnetic phenomena, as developed by Maxwell, Hertz and Lorentz, should apply equally relative to moving or to stationary bodies. Yet these equations seemed to involve intrinsically the constancy of the speed of light. As Einstein explained in 1922,
“the fact that these equations work also in the moving ordinary system leads to the invariance of the speed of light. However, it doesn’t agree with the laws about the addition of velocities, which we knew in force physics. Why do these two things conflict with each other? I felt like I was hitting a dead end of extreme difficulty.” 251
Likewise, he later reiterated, that in his efforts to attain an effective approach to electrodynamics, “The difficulty that had to be overcome was in the constancy of the velocity of light in vacuum which I first thought I would have to give up.”252
The concept of the invariance of the speed of light contradicted the basic law that all velocities vary according to a simple addition rule. This rule was practically a mathematical statement of the relativity principle. In hindsight, Einstein explained: “the constancy of light propagation seems to conflict with the classical principle of relativity, according to which the velocity of a ray of light assumes different values in the moving system according to the direction of the ray.”253 For example, if a person walks at a speed w inside a railway carriage (reference system) traveling uniformly at a velocity v relative
bottom of p.263


to the ground (a second reference system), then this person moves relative to the ground with a velocity of v + w. Since the behavior of all bodies on the train (or “moving reference frame”) are affected in the same way, that is, by the addition of a velocity v, then they all behave in the same way with respect to one another, so that “the laws of physics” hold in the same way relative to the train and to the embankment. Because the invariance of light velocity contradicted the rule of addition of velocities, the relativity of motion and the constant speed of light were “apparently irreconcilable.” 254
Struggling to solve the problem, to modify parts of Lorentz’s theory, Einstein felt that there was scarcely any secure theoretical foundation on which to build. Owing to the works of Max Planck and others, Einstein believed that neither mechanics nor electrodynamics could be exactly valid.255 He had also lost his early faith in the exact validity of Maxwell’s equations. Einstein had found that Maxwell’s theory gave incorrect predictions for the fluctuations of radiation pressure and the motions of tiny bodies exposed to radiation: “In my view, one could not get around ascribing to radiation an atomistic structure which, of course, does not fit into the frame of Maxwell’s theory…”256 Thus he “had already found that Maxwell’s theory did not account for the microstructure of radiation and could not therefore have general validity.”257 Accordingly, optics could hardly provide a secure foundation. The corpuscular behavior of light and electromagnetic radiation in some experimental areas didn’t harmonize with the well-known wavelike effects of light, such as interference and polarization. In sum, “Einstein saw that his efforts began to endanger the supposedly impregnable foundations of his science.”258
Failing to devise an electrodynamics on the basis of constructive efforts, that is, based on other theories and hypotheses about the constitution of matter and radiation, Einstein then hoped to find some principle that would at least outline the general form of the theory:
bottom of p.264


“By and by I despaired of the possibility of discovering the true laws by means of constructive efforts based on known facts. The longer I tried, the more I came to the conviction that only the discovery of a universal formal principle could lead us to assured results. The example I saw before me was thermodynamics. The general principle there was given in the theorem: the laws of nature are such that it is impossible to construct a perpetuum mobile (of the first and second kind). How, then, could such a universal principle be found?”259
In 1916 Einstein told an interviewer that as his original ideas began to arise, “I was plagued by all sorts of nervous conflicts; I went around confused for weeks,” as if in a “stage of stupefaction.”260 In 1932 he recalled that “after seven years of searching in vain, the desire to discover and overcome this generated a state of mental tension in me.”261 From his comments, one early biographer gathered that Einstein’s mind “ruminated” over his relativity thoughts even “while he sat alone undisturbed in the work room of the patent office.”262 Reportedly,
“To Besso were poured out all the disappointments and anguish of frustrated moments, of the travail of research. He would cry in despair to him: “It is no use to try farther; I must give it up.” Then Besso, with an understanding so great and a patience so infinite, would calm the troubled thought.”263
Besso was encouraging. Regarding Einstein’s ideas, he liked to say “If they are roses, they will bloom.”264 Another biographer, who in 1962 interviewed Einstein’s son, Hans Albert, recounted:
“He worked on and on through 1904. One puzzle led to another. His brain was on fire. His body was exhausted. He could not eat. He could not sleep. The speed of light never varies. When people spoke to him, he did not hear. He wandered about in a daze. At times he wondered if this was the way to insanity.”265
Einstein tried to divide his time between his investigations, his work, and his family. But his theoretical thoughts overran him. He would take his son in “a baby carriage around the streets of Bern. When he felt that he had
bottom of p.265


fulfilled his fatherly duties, he would hurry home to pore over problems, which, like an overwhelming passion, possessed him, never letting him rest.”266 He had become obsessed with the problem, it drove him to despair:
“Now he experienced the full tragedy of the researcher: his toil was not successful, yet he could not leave it, he could not stop his ruminations; Einstein feared for his health. And only with an intense exertion of the will did he succeed in freeing himself from these thoughts, in compelling himself to think about that no more.”267
Reportedly, all of his efforts “to obtain a theory consistent with the experimental facts had failed.”268 Einstein forced himself to stop working on the problem.
The Eagle and the Sparrow
In October of 1904 Solovine moved back to Bern. And, the “abominable creature,” Habicht, responded to Einstein’s “enraged” scolding by occasionally spending time in Bern.269 Thus, the trio could resume meetings of their Academy. It was a rewarding distraction for Einstein from his obsessive thoughts about electrodynamics.
In early 1905, Solovine, Einstein, and Habicht read Hume’s Treatise of Human Nature, “in a very good German edition.”270 Reportedly, “What Einstein liked most about Hume was the unsurpassable clarity of his presentation and his avoidance of any ambiguities intended to give an impression of profundity.”271 Einstein later reminisced that above all other subjects, they busied themselves with discussions of Hume.272 Likewise, Solovine later recalled that, in particular, “we discussed for weeks David Hume’s singularly incisive critique of the notions of substance and causality.”273 Regarding the notion of causality, Hume argued that although certain happenings seem to regularly follow one another, we can never know whether they are really
bottom of p.266


necessarily connected.274 Events appear joined in a temporal sequence, as we experience them, but we do not know if one event is necessarily the cause of its successor. Thus the fundamental notion of causality is a human conception; it is not justified even by our scientific knowledge. For Einstein, this reading of Hume was “of considerable influence upon my development—alongside Poincaré and Mach.”275 As with Kant, Einstein felt that he could “perfectly understand” Hume.276 In hindsight, he wrote that “Hume’s clear message seemed crushing”: human sensations are the only source of knowledge, they are linked only by expectations and habit, and they cannot lead to real laws of nature.277 Einstein became convinced that
“All concepts, even those which are closest to experience, are from the point of view of logic freely chosen conventions, just as is the case with the concept of causality, with which this problematic concerned itself in the first instance.”278
Poincaré had also drawn attention to the extent to which certain fundamental concepts in science are conventions. Given Hume’s formulation, Einstein increasingly overcame a teaching of Kant: that certain fundamental notions are indispensable, as they are not concepts, they are not based on experience.279 Thus Einstein felt “a special kinship” with Hume.280
Kant had written his Critique of Pure Reason partly as a reply to Hume’s Treatise. Einstein happened to read these works in reverse, so in his case, it was Hume’s earlier work that undermined Kant’s later arguments. Hume showed a “more sound instinct” than Kant.281 Einstein readily showed how impressed he was by Hume. In early March 1905, Solovine proposed that instead of holding an Academic meeting they could attend an upcoming violin concerto by a famous Bohemian string quartet. Despite Einstein’s love of music, he replied that they should not, that it was much preferable
bottom of p.267


to read Hume.282 On Friday March 17, when the trio was to hold their meeting at Solovine’s apartment, Solovine suddenly could not resist but attend the concert on his own.283 He left the door open so that Einstein and Habicht could meet at his place nevertheless. Einstein and the “mischievous” Habicht were outraged.284 They decided to smoke as many cigars as they could in his apartment, with closed windows, in order to saturate it with the smell that Solovine despised. Then they smeared the ashes and cigar-butts on Solovine’s bed, table, chairs, forks, teacups, teakettle, and more. When Solovine returned he could not stand it. The next day Einstein chastised him for not fulfilling his academic duty:
“You miserable subordinate, how! you have the audacity to neglect an academic meeting to go hear violins? Barbarian, Boetian, if ever you allow yourself again such an outrage, you will be excluded and shamefully chased from the Academy!”
That night their discussion of Hume extended late until one hour before sunrise.
Einstein also continued to work on physics, while he avoided the problems of electrodynamics. He avoided the puzzles about the speed of light and the motion of reference frames. But nonetheless, he still thought about electrons and light. According to one writer (who at best may have conveyed a third-person account), Einstein and Besso discussed as follows:
“One thing baffles me,” said Besso one night when they had been theorizing on the action of electrons—the smallest quantity of electric charge known to exist independently. “A metal plate exposed to a beam of light gives forth a shower of negatively charged particles. Right?” “Ja,” said Einstein, nodding amiably. “Why?” asked Besso. “Ach, that I cannot explain.”
For days Einstein pondered the question put to him by Besso. Finally he told his friend. “I think I have an explanation for your electrons, but first you’ll have to accept the idea of light as composed of individual particles of energy…”285
bottom of p.268


Einstein argued that processes of the emission and absorption of radiation can be best understood if radiation is assumed to have a corpuscular nature, that is, if light is made up of particles. Einstein told Besso of his “heuristic viewpoint,” and Besso “immediately recognized it as a discovery of the utmost importance and of the greatest consequence.”286 Reportedly, Besso said “It may take time to absorb that idea,” that light consists of particles.287 It became their main subject of discussion.288 Hence, in March of 1905, Einstein completed a paper about the production and transformation of light.289 Still, Einstein could not explain how or why, if indeed light consists of particles, one yet observes experimentally properties such as interference, which seem to suggest wave motions in a medium. Moreover, he still espoused the traditional hypothesis that the speed of light, like the speed of waves, is independent of the speed of its source. Virtually all physicists believed that light consisted of waves in the ether. Thus the irreverent idea of light quanta seemed “very revolutionary.”290
Einstein also managed to complete works that analyzed matter in terms of corpuscular conceptions. At the end of April, he finished a paper on determining the size of molecules, which he then submitted as a doctoral dissertation to the University of Zurich. He dedicated this work to his friend Marcel Grossmann, in appreciation for his help at the Polytechnic, and, towards getting the job at the patent office. Very soon Einstein also submitted yet another paper to the Annalen der Physik, explaining the motions of small particles suspended in stationary liquids as resulting from microscopic collisions with molecules. Following the kinetic-molecular theory of heat, Einstein made predictions about the motion of small particles which, in turn, could serve to provide experimental evidence of the theory of molecules and atoms. Einstein’s departure from the problems of electrodynamics was hence very productive.
Nevertheless, early in the Spring of 1905, Einstein still had not solved the problems of light and relative motion. According to a biographer
bottom of p.269


(who once interviewed Einstein’s son), “Frustration drove him out to wander the farm lands around Bern. He took time off from the office. Mileva helped him solve certain mathematical problems, but nobody could assist with the creative work, the flow of fresh ideas.”291 He had been trying to reformulate Lorentz’s electrodynamics since mid-1904: “Expecting that I had to somehow modify Lorentz’s theory, I had spent almost a year thinking in vain, and I couldn’t help but think that this puzzle is not easily solved.”292 After “ten years of reflection,”293 including seven years of focused efforts, Einstein had not solved his puzzles on the optics of moving bodies and electrodynamics.
An account of those days was published in 1930 by Einstein’s son-in-law, Rudolf Kaiser, writing under a pseudonym. Einstein wrote a brief Preface to the completed biography, noting that “The author of this book is one who knows me rather intimately,” and that “I found the facts of the book duly accurate.” Kaiser described Einstein’s conceptual struggles:
“As a man possessed, he was carried away by these most difficult problems of theoretical physics, and talking about them helped more than silence. Going home from his duties he discussed his ideas and investigations with his friend Besso.”
Years later, Einstein characterized Besso as follows: “he is brilliant in discussions. There he aims to bridge conflicts and discrepancies. This and his talent to penetrate into the thoughts of others make him a fertile critic.”294
In early May 1905, Einstein lived at 49 Kramgasse, at the heart of old downtown, east of the patent office building (at the corner of Genfergasse and Speichergasse). Besso sometimes walked Einstein home after work. Kayser recounts how Einstein informed Besso of his attempts to solve his theoretical problem:
“If he felt that he was nearing a solution of his problems, he would tell his friend—his own eyes glowing—that the success of his efforts was at hand. But the next day he would merely inform him sadly and gently that all his attempts of the past were wrong. Through many long years of hope and disappointment Albert carried on his attempts…”295
bottom of p.270


Einstein had fought many battles without success. Finally, one day he decided to take the entire problem to Besso, to discuss it at length. Perhaps Besso’s sharp attention to “petty” things would be helpful.296 In 1922, in a lecture delivered in Kyoto, Japan, Einstein recounted:
“But by chance, a friend of mine in Bern helped me out. It was a beautiful day when I visited him, saying ‘Lately, I have one problem that I cannot figure out in any way. Today I’ve brought you that war.’ ”297
Reportedly, Einstein “admitted that he had run up against a complete mental block.”298 So he shared many aspects of the problem, “And I tried various discussions with him.”299 Years later, Besso used the following analogy: “Einstein, the eagle, took me, the sparrow, under his wings into the heights, and then there I fluttered yet a little farther above.”300 Einstein recalled: “By that, suddenly I came up with a clear idea.”301 During so many years, Einstein had pursued and “abandoned many fruitless attempts, ‘until at last it came to me that time was suspect!’”302
In 1916, a psychologist studying scientific creativity, Max Wertheimer, interviewed Einstein at length regarding the thoughts that originally led to the solution of his conundrums on relative motion. Apparently, Einstein realized that the phenomena of motion in question (the phenomena of electrodynamics and light) necessarily involve a tacit measurement of time. Reportedly, he asked himself: “Do I see clearly, the relation, the inner connection between the two, between the measurement of time and that of movement? Is it clear to me how the measurement of time works in such a situation?”303 We can infer more specifically that the concept of simultaneity emerged as having crucial importance. Wertheimer reported “characteristic remarks” of Einstein:
“the discovery that the crucial point, the solution, lay in the concept of time, more particularly in that of simultaneity …. The very moment he saw the gap, and realized the relevance of simultaneity, he knew this to be the crucial point for the solution.”304
bottom of p.271


Presumably, “the gap” was between traditional basic concepts and physical experience.
Did Einstein find the solution to his problem right then in the company of Besso? Apparently not, further evidence suggests that what he found right then was not quite the solution, but something about the essence of the problem, the “key” to its solution: “When he held the key, with which he was to open the closed door, in his hand, he despaired, and said to his friend ‘I’m going to give it up.’”305 Another biographer echoed: “It’s no use,” he told Besso. “I’m on the wrong track.”306 Apparently Einstein then left Besso, perhaps frustrated, but having found at least the crux of the problem, something about the relationship between the kinematic concepts of time and motion, as we will see.
Back home, Einstein likely analyzed the problem further that evening. He believed that “A notion acquires its right to existence uniquely by its clear and univocal enchainment with events, with respect to physical experiences.”307 It was precisely for that reason that already he had long rejected the notion of “absolute velocity,” that is, because its “univocal connection with experiences showed itself to be impossible.”308 Likewise, how was the notion of simultaneity connected with experience? As reported by Max Wertheimer, Einstein then “said to himself”:
“If two events occur in one place, I understand clearly what simultaneity means. For example, I see these two balls hit the identical goal at the same time. But . . . am I really clear about what simultaneity means when it refers to events in two different places? What does it mean to say that this event occurred in my room at the same time as another event in some distant place? Surely I can use the concept of simultaneity for different places in the same way as for one and the same place—but can I? Is it as clear to me in the former as it is in the latter case? . . . It is not!” 309
bottom of p.272


According to Wertheimer, Einstein proceeded to analyze the question along the following lines. He asked himself how one can determine whether two lightning bolts strike distant places at the same time.310 By conceiving of a feasible physical procedure, one would be able to ascribe a practical meaning, a definition, for the word “simultaneity.”
“How could the notion of the simultaneity of distant events be connected with experience? How was the notion of a rigid body connected with experience? How was the geometric shape of a moving body connected with experience? Perhaps Einstein realized that the latter questions depend on the former: to determine the length of a body we need to know the simultaneous location of its extremities. One would need to somehow ascertain the simultaneity of distant events in order to in any way measure a moving length. Moreover, measurements of velocities depend on measurements of lengths and times. Whether or not the speed of light varies relative to a moving system depends precisely on how one measures that speed. Later Einstein recalled: “Only after groping for years did I notice that the difficulty rests on the arbitrariness of the fundamental kinematic concepts.”311 Finally some clarity, but no solution.
At some point that night, Einstein went to sleep. Incidentally, he once explained that he routinely stopped thinking about problems at bed time: “I break off when I want, and banish all difficulties when the time to sleep arrives. A thoughtful dream-work, as in the case of artists, whereby poets and composers weave the day into the night, is foreign to me.”312 But maybe that one night was different. One biographer claimed that “That night when he went to bed, he lay awake tossing and turning.”313
bottom of p.273


Another biographer, his other son-in-law, Dimitri Marianoff recounted what Einstein once replied when asked how he had arrived at his theory:
“He said that one night he had gone to bed with a discouragement of such black depths that no argument would pierce it. “When one’s thought falls into despair, nothing serves him any longer, not his hours of work, not his past successes—nothing. All reassurance is gone. It is finished, I told myself, it is useless. There are no results. I must give it up.”314
But the pieces of the puzzle were falling into place. According to Marianoff, Einstein’s thoughts proceeded “In vision,” as the “underlying unity of size, structure, distance, time, space slowly fell piece by piece, like a monolithic picture puzzle, into place…”
If thinking during sleep hours was unusual, thinking in the morning was another matter. Jakob Ehrat, his friend from the Polytechnic, reported what Einstein once told him: “One morning on awakening, as I was sitting up in bed, the breakthrough thought came to me.” He referred to the notion that two events that are simultaneous for one observer are not necessarily simultaneous for another.315 Years later, another friend too recalled that “Einstein said his basic discovery came on waking up one morning, when he suddenly saw the idea. This had been going around and around at the back of his mind for years, and suddenly it wanted to thrust itself forward into his conscious mind.”316
Einstein realized that if one were to determine the simultaneity of distant events by employing a definite measurement procedure, then that procedure might yield different results when applied in systems in relative motion. For example, a person aboard a moving train could perceive two lightning bolts in a different sequence from what is observed by someone on the ground.317 This would serve to formulate a new concept of simultaneity, a relative concept. Einstein could reject the traditional notion of “absolute simultaneity” because it seemed to be impossible to connect it univocally with experience.318 Einstein believed that in physics there should be “no notion of which its use is a priori necessary or justified.”319 Even the fundamental notion of absolute time was susceptible of being modified.
bottom of p.274


Einstein believed that physical concepts should acquire meaning by their connections to definite empirical relations. Kinematic concepts would be meaningful insofar as they related to experience. Notions such as distance, speed, and acceleration, should all be defined by the relations among definite material things, such as rigid rods and synchronized clocks. Length could be defined by measuring sticks. Instants of time could be defined merely as the indications of a pointer on a clock-face. If moving observers disagreed over the simultaneity of distant events, then they would disagree accordingly about the lengths of moving bodies. They would also disagree over other related measurements. But such disagreements could be treated systematically and consistently. In this way, now “length contraction” would not be an artificial and independent hypothesis at all, it emerged as a necessary consequence of specific measurement procedures. Moreover, observers would disagree over the interval of time between two events. Therefore, they could obtain the same measurement for the speed of a single ray of light, even while the observers move in opposite directions. The measured speed of light could thus be independent of the motion of reference systems.
As suggested by several sources, Einstein’s conceptual breakthrough was thus the idea of the relativity of simultaneity. For example, his childhood mentor and friend, Max Talmud, emphasized that, “The discovery that the simultaneity of distant events is relative was the origin of the relativity theory.”320 If we accept this proposition then we may conjecture that Ehrat’s quotation indeed referred to the very morning after Einstein had his productive conversation with Besso. Then that next day, as he recalled in 1922, Einstein promptly informed his friend:
“So the next day I came back to him again and approached him, without any greetings: “Thank you. I have completely solved my problem.” My solution was about the very concept of time. Thus time is not absolutely defined, and there is an inseparable relationship between time and signal speed. That previous extraordinary difficulty was solved completely by that, for the first time.”321
bottom of p.275


If we continue to trust that all the recollections here quoted describe correctly the sequence of events, it appears that that same “next day” when Einstein saw Besso again, was a work day at the Patent Office, as Kaiser’s account continues:
“…the next day it was in the greatest excitement that he took up his duties at the office. He could apply himself to the dull routine only with effort. Feverishly he whispered to his friend that now at last he was on the right track. He made the revolutionary discovery that the traditional conception of the absolute character of simultaneity was a mere prejudice, and that the velocity of light was independent of the motion of coördinate systems.”322
Perhaps Einstein “whispered” his findings because he didn’t want to upset his supervisor at work, or perhaps he was not ready to share his ideas just like other young innovators, we don’t know. Other colleagues such as Dr. Sauter did not enjoy the intimate friendship that Einstein shared with Besso. What matters is that Einstein suddenly and finally found a solution. In 1924 he recalled:
“After seven years of reflection in vain (1898-1905), the solution came to me suddenly with the thought that our concepts and laws of space and time can only claim validity insofar as they stand in a clear relation to our experiences; and that experience could well lead to alteration of these concepts and laws. By a revision of the concept of simultaneity into a more malleable form, I thus arrived at the special theory of relativity.”323
Finally Einstein moved decisively forward, he took “the step,” as he called it years later.324 Yet regarding his progress towards his new conception,
bottom of p.276


Einstein once explained that his thoughts had not moved directly, step by step, to the solution, but by a tortuous path. He commented that “It is only at the last that order seems at all possible in a problem.”325
In 1915 Einstein commented that “a great influence” on his labors was effected by his readings of “Mach, and ever much more Hume, whose treatise on [human] understanding I studied with fervor and admiration shortly before figuring out the theory of relativity. It is very much possible that without these philosophical studies I would not have arrived at the solution.”326 Late in life Einstein reiterated that influential roles of Hume and Mach. He wrote that all efforts to resolve the problematic relationship between electromagnetism and mechanics had to contend with the great difficulty that
“the axiom of the absolute character of time, viz., of simultaneity, unrecognizedly was anchored in the unconscious. Clearly to recognize this axiom and its arbitrary character really implies already the solution of the problem. The type of critical reasoning which was required for the discovery of this central point was decisively furthered, in my case, especially by the reading of David Hume’s and Ernst Mach’s philosophical writings.”327
Accordingly, when someone once asked Einstein how he had come to formulate the theory of relativity, Einstein replied: “By refusing to accept an axiom.”328
He promptly shared his new ideas with Besso. According to a third-person account, they discussed the subject of simultaneity as follows:
“ ‘Did it ever occur to you that many events that seem simultaneous are not truly so?’ Einstein asked Besso one evening as they sat munching Swiss chocolate. ‘This morning as I left the house,’ said Besso, ‘a factory whistle emitted a blast. Are you going to tell me that the whistle blast and my departure from the house were not simultaneous?’ ‘The sound of the whistle came from some distance away,’ Einstein pointed out. ‘So it took an interval of time—although an extremely short one, I’ll admit—for the sound to reach your ears. Therefore the whistle blast and your exit were not exactly simultaneous.’ Besso rubbed his chin thoughtfully. ‘What you’re really saying is that the idea of simultaneity depends on velocity, time, and the position of the observer of the event.’ ‘Precisely!’ exclaimed Einstein, pleased that Besso grasped the idea so quickly.”329
bottom of p.277


Traditionally, physicists had assumed that in principle all observers should be able to agree on the time of any given event. Thus for two reference frames in relative motion, the time of an event according to observers on each frame would be the same: (t’ = t). Likewise, such observers should agree on the duration of any physical process: (t2’= t1’) = (t2 = t1). These notions (or ‘transformation equations’) seemed so natural, and necessarily true, that they were nearly everywhere employed tacitly. Problems in electrodynamics had brought them to some physicists’ attention. Einstein hence realized that if observers in relative motion disagree about the simultaneity of distant events they could accordingly disagree about the precise time of events and about durations. So he abandoned the traditional conception of time, dismissing it as arbitrary.
In its place, Einstein posited a concept of time that could be established by physical procedures. Like Poincaré, he knew that Lorentz’s local time would actually be the measures of time given by moving clocks synchronized by the exchange of light signals. Einstein decided that this procedure for establishing time could be used systematically to establish the time in all non-accelerated material systems. It occurred to him that Lorentz’s artificial time could be defined as the physical time, pure and simple. It alone seemed meaningful:
“Surprisingly, it became evident that to overcome the aforementioned difficulties, it was only necessary to make the concept of time sufficiently precise. It only required the knowledge that the auxiliary quantity introduced by Lorentz, which he called “local time,” can be defined simply as the “time.” Holding firmly to this definition of time, the fundamental equations of Lorentz’s theory then agree with the principle of relativity if only the above mentioned transformation equations are replaced with such as correspond to the new concept of time.”330
bottom of p.278


Einstein had concluded that idea of a universal time was just an unjustified assumption. By abandoning that concept, he then could apply Lorentz’s equations in any non-accelerated systems. Letting t describe the time of an event as observed from one system, then the equation that expressed the time of the same event with respect to another system was:

Here, x is the position of the event in the first system, and v is the relative speed between the two systems. Despite its seeming complexity, this equation gave systematic and consistent results. Accordingly, several other transformation equations would serve to relate measurements of position, length, velocities, and so forth, between reference systems.
Maxwell’s equations did not change form when reformulated with the exact transformations that stemmed from Lorentz’s works. Hence Maxwell’s equations were valid in all inertial systems, they constituted “laws” of physics. By extending the validity of these transformations to kinematics, and thus to all branches of physics that depend on kinematics, Einstein found the formal principle analogous to that which underlay thermodynamics.331 The theory of heat and energy was based on the principle of the non-existence of a perpetuum mobile. That principle restricted the possible laws of physics. Likewise, Einstein realized that the laws of mechanics, like the laws of electricity and magnetism, should be invariant with respect to Lorentzian transformations. Since the laws of mechanics were not invariant with respect to these transformations, Einstein proceeded to modify such laws.
When transforming the length of a rigid rod stationed in one system into its determination with respect to a system moving relative to the first, the latter length would be shorter than the former. Moreover, the effect would be reciprocal: observers in one system would judge that the meter-sticks on
bottom of p.279


the other system were contracted, whereas observers on the latter would judge that instead it is the meter-sticks in the former system that are contracted. Thus Einstein found that the “hypothesis” of length contraction, which had earlier been introduced as an artificial and independent assumption, now emerged “as a compelling consequence” of the new approach.332
Likewise, the traditional “law” of the addition of velocities could be replaced with a proposition based on the relations between measured distances and times. It was not a priori necessary that the new “addition” rule would be the same as the traditional one, and Einstein found that indeed it was not. The new rule would account for why certain optical experiments didn’t seem intelligible in terms of the traditional “law.” Thus Einstein proceeded to change the classical laws of kinematics and dynamics.
Alongside the critical outlook that enabled Einstein to reject traditional concepts, he also took creative liberties. On the one hand, he had found that the fundamental categories that Kant had construed as being unchangeable, could actually be modified. On the other hand, he had found a way in which he could contribute innovations into the so-called laws of physics. “This had come from the inventive element of human reason that Kant had written about. Einstein had proved his creativity. He was ecstatic.”333
On Sunday the 14th of May 1905, Hans Albert Einstein had his first birthday. At around that time, the Einstein-Maric family moved from the heart of downtown Bern to a residence just a few blocks away from Michele and Anna Besso. Einstein and Besso began to daily walk back from the patent office to their homes together, and continued to discuss science. In long conversations, Besso played “the role of a critical disbeliever,” he “defended Newton’s recognized concepts of space and time,” which he justified by appealing to Mach’s arguments.334 Despite Mach’s rejection of the notions of absolute space and time, one might yet uphold a relative conception of space and time that agreed with Newton’s mechanics. But Einstein upheld his new and radical concepts with conviction. For Einstein, their “conversations on the way home had an incomparable charm.”335
bottom of p.280


Decades later, a writer (who interviewed Hans Albert) narrated a conversation that allegedly transpired between Einstein, Besso and Maric. Reportedly, Einstein argued:
“Nothing except light is ever the same for two consecutive seconds,” he told Mileva and Besso one night when they sat talking in the apartment. “It always journeys at the same speed.” “In space, yes; but how about light from that?” Mileva asked, glancing toward a lamp. “Whether it comes from a candle, a lamp, an electric-light bulb, or a star, it is unalterable.” “Suppose,” asked Besso, “that you could put light aboard a rocket. Wouldn’t its velocity be increased?” Einstein drummed his fingers on the arm of the wooden rocker in which he sat. “It might be expected that the velocity of the light traveling from the source in the rocket would be 186,000 miles per second, plus the velocity of the body on which it traveled. But nothing can exceed the speed of light. I hope to prove that it remains independent of the system in which it travels.”336
Still in May, there were plenty of news to tell another good friend. Einstein wrote to Habicht to tell him about his latest papers and more. As usual, he bantered:
“So what are you up to, you frozen whale, you smoked, dried canned piece of soul, or whatever else I would like to hurl at your head, filled as I am with 70% anger and 30% pity! You have only the latter 30% to thank for my not having sent you a can full of minced onions and garlic after you so cravenly did not show up on Easter. But why have you not sent me your dissertation? Don’t you know that I am one of the 11/2 fellows who would read it with interest and pleasure, you wretched man? I promise you four papers in return. … The fourth paper is currently a rough draft and is an electrodynamics of moving bodies which employs a modification of the theory of space and time; the purely kinematic part of this paper will surely interest you.”337
bottom of p.281


Einstein outlined his paper: the “Kinematic Part” would precede the “Electrodynamic Part,” to solve problems in the latter. He devised a new kinematics based on the realization that Lorentz’s notion of local time could be rigorously applied to physics in general.
Einstein was impressed by the agreement between his kinematic approach and Lorentz’s equations. Aspects of Lorentz’s theory, such as length contraction and the electromotive force, which previously seemed to be independent hypotheses, now emerged as consequences of Einstein’s approach. Such effects stemmed from the relativity of motion and of simultaneity. His account corresponded better with the symmetries evidenced by electrodynamic experiments, because it did not pick out any one reference frame as special, as being truly at rest. Thus the concept of a universal stationary ether seemed superfluous. “Only the introduction of a light ether as the carrier of electric and magnetic forces” did not harmonize with his conception, where instead, “electromagnetic fields appear here not as the state of any matter but as independently existing things,” as he had anticipated.338 Yet there remained a fundamental aspect of Lorentz’s ether conception, namely, the constancy of the speed of light. Following Lorentz, Einstein had assumed that light speed is constant, at least relative to a given reference frame, and that it is independent of the speed of its source. In 1912, Einstein explained:
“it is impossible to base a theory of the transformation laws of space and time on the principle of relativity alone. As we know, this is connected with the relativity of the concepts of “simultaneity” and “shape of moving bodies.” To fill this gap, I introduced the principle of the constancy of the velocity of light, which I borrowed from H. A. Lorentz’s theory of the stationary luminiferous ether....”339
Accordingly, in a long manuscript of 1912-14, Einstein emphasized the connection between the light principle and Lorentz’s theory. He remarked that “this principle is demanded by Lorentz’s theory,” and later he even called it: “the principle of the constancy of the velocity of light (Lorentz’s theory).”340
bottom of p.282


The ideas that Einstein analyzed since the mid-1890s included the concepts of relativity and of the velocity of light; but only in 1905, having found a solution in his analysis of basic kinematic concepts, did the two “principles” emerge as a foundation for his novel theory.
Once Einstein had formulated his general sketch of his paper on kinematics and electrodynamics, it took him about five weeks to complete the article. One biographer echoed: “The transfer of the broad concept of the theory to its logical mathematical progression on paper took five weeks of sapping work.”343 At the end of his paper, Einstein included the following acknowledgment: “In conclusion I wish to say that in working at the problem here dealt with I have had the loyal assistance of my friend and colleague M. Besso, and that I am indebted to him for several valuable suggestions.”344
Einstein worked himself to exhaustion. He recalled later that he “suffered a collapse …. The upheaval was violent.” A biographer, who interviewed his son Hans Albert, recounted that when he finished his paper, “Benumbed, groggy, and terribly tired, he went to bed…”346 Likewise, another third-person account recounted: “When it was over, Einstein’s body buckled and he went to bed for two weeks. Mileva checked the article again and again, then mailed it. ‘It’s a very beautiful piece of work,’ she told her husband.”347
Then “He was ill for fifteen days.”348 Reportedly, “For two weeks Einstein, worn out by his exertions, ate very little and slept almost not at all. The slightest effort tired him.”349 Back at the office, Einstein told his senior colleague, Josef Sauter, about his latest work. Having finished his paper, he told Sauter that he was overjoyed to find, after all, that his results were perfectly compatible with the equations that Lorentz had ascertained. Decades later, Sauter recalled that Einstein said: “I cannot express the intensity of my joy.”350
bottom of p.284


Einstein found that he could formulate his kinematics of clocks, rigid bodies and electromagnetic processes on the basis of two key assumptions. Allegedly, according to a third-person account, “As he continued to pursue the subject of simultaneity, Einstein began to use the word ‘relativity’ more and more.”341 Rather than having the principle of relativity be valid as a byproduct of several compensating effects, as in Lorentz’s theory, he chose to raise the status of the principle to a postulate. That is, he chose to posit it as a fundamental assumption. Likewise, he postulated the constancy of the speed of light in an arbitrary inertial system. From those two postulates, along with physical hypotheses about the behavior of clocks, rods, and light, Einstein proceeded to derive the equations of his kinematics.
Thus Einstein chose to formulate his new approach as a structured series of mathematical propositions deduced from fundamental axioms. In so doing he followed an ancient tradition: the axiomatic presentation of mathematical knowledge, as carried out throughout the centuries by mathematicians and scientists such as Euclid, Newton, and more recently, Hertz. This approach is distinguished by its logical elegance and pedagogical usefulness, yet it often seems to suggest, incorrectly, that the axioms on which a theory is based constituted also the original sources of the theory. In the case of Einstein’s kinematics, accordingly, one should not confuse the axioms with its roots. In a series of interviews, the psychologist Max Wertheimer documented Einstein’s recollections in this regard, noting that: “The axioms were not the beginning but the outcome of what was going on …. The axioms were only a matter of later formulation—after the real thing, the main discovery had happened.” Underscoring that point, Wertheimer added:
“I wish to report some characteristic remarks of Einstein himself. Before the discovery that the crucial point, the solution, lay in the concept of time, more particularly in that of simultaneity, axioms played no role in the thought process—of this Einstein is sure. (The very moment he saw the gap, and realized the relevance of simultaneity, he knew this to be the crucial point for the solution.) But even afterward, in the final five weeks, it was not the axioms that came first. “No really productive man thinks in such a paper fashion,” said Einstein.”342
bottom of p.283


And, Solovine remarked that Einstein’s work benefited from his good life at home in the company of Mileva: “I am convinced that her influence was beneficial, in the environment and affection and in allowing him to work peacefully. I cannot forget that the memoir On the Electrodynamics of Moving Bodies, which established his reputation, was published in 1905, where a perfect harmony reigned between them.”351
Creative Logical Sins
Einstein’s contribution to kinematics arose suddenly in the context of his prolonged labors to solve problems in other fields of physics. In 1922, in a reception at Paris, someone asked him about special relativity: “Did it come to you all at once, or did it take you a long time?” To which he replied: “It came to me all at once, after having thought about it for my whole life.”352 Accordingly, Einstein once explained to his biographer, Carl Seelig, that there transpired
“five to six weeks between the conception of the idea for the special theory and the completion of the relevant publication. But it would hardly be correct to consider this as a birthday, because earlier the arguments and building blocks were being prepared over a period of years, although without bringing about the fundamental decision.”353
Was the formulation of the special theory of relativity a discovery or an invention? Nowadays many writers call it a “discovery.”354 But throughout his life, Einstein emphasized the importance of invention, when characterizing his theoretical contributions. Alexander Moszkowski was very interested in scientific discovery but was shocked to hear Einstein argue: “The use of the word ‘discovery’ in itself is to be deprecated. For discovery is equivalent to becoming aware of a thing which is already formed…. Discovery is really not a creative act.”355 By contrast, the formulation of the special theory of relativity was a creative act. But that’s not to say that Einstein
bottom of p.285


invented concepts such as the principle of relativity, local time, the constancy of the speed of light, or the contraction of lengths. Such concepts had already been conceived and developed by physicists such as Lorentz and Poincaré. It was thanks to the labors of Lorentz, above all, that Einstein and many other physicists were able to pursue electrodynamics along definite lines. Above all other physicists, Einstein loved Lorentz.356 Accordingly, late in life he complained: “People do not realize how great was the influence of Lorentz on the development of physics. We cannot imagine how it would have gone had not Lorentz made so many great contributions.”357
Thus Einstein’s contribution was scarcely the conception of the basic concepts in electrodynamics. Instead, what Einstein invented was a new way of arranging such concepts in relation to one another. In so doing, he changed the meaning of such concepts. He elaborated: “Invention occurs here as a constructive act. This does not, therefore, constitute what is essentially original in the matter, but the creation of a method of thought to arrive at a logically coherent system…”358
Reportedly, in conversation, Einstein once commented that there existed “a definite connection between the knowledge that he gained at the Patent Office and the theoretical results that appeared at the same time as products of his intensive thought.” At the patent office, as in his father’s business and in the laboratories of the Zurich Polytechnikum, Einstein maintained contact with innovative technologies. Haller, the Director of the Patent Office “taught them [the patent clerks] to think sharply and logically, and to select every word in its most exact sense.”360 He encouraged Einstein to cultivate critical scrutiny and a precise clarity of expression, as Einstein recalled that “This man [Haller] taught me how to express myself correctly; he was stricter than my father.”361 And at the patent office, Einstein could discreetly discuss his theoretical investigations with Besso.
bottom of p.286


Besso himself tried to ascertain the origin of Einstein’s insights. Rather than drawing any connection with modern technologies, Besso believed that the distinctive roots of Einstein’s creativity lay in a critical philosophical outlook. In 1947, at the age of seventy-four, Besso wrote to Einstein to ask: “Is it true” that his having introduced the young Einstein to Mach’s writings exerted such an influence in the developing student that it “turned his attention to observable things—even maybe indirectly, to ‘clocks and measuring rods?’”362
Einstein soon replied, acknowledging that Besso had introduced him to Mach’s writings while he was a student at Zurich, and that Mach’s influence on his intellectual development in general had been great. But more specifically, he added that “frankly,” he could “not clearly see” any direct influence of Mach on his work of 1905. By contrast, Einstein claimed to have been influenced directly by Hume: “As far as I am aware of, the immediate influence of D. Hume upon me was greater. I read this together with Conrad Habicht and Solovine in Bern.”363 Einstein reminisced that in their evening discussions, the reading of Hume’s Treatise had been a major influence on him “—alongside Poincaré and Mach.”364
Einstein’s approach to physics was consonant with Hume’s arguments that exact scientific laws cannot be induced from experience, and that the truth of generalizations from experience is often unjustifiably asserted owing to merely habitual expectations. The universality of time appeared as just such an unjustified concept. In hindsight, Einstein commented that “Hume saw clearly that certain concepts, as for example that of causality, cannot be deduced from the material of experience by logical methods.”365 Likewise, Einstein realized that he could not deduce the concept of absolute time from the material of experience. Consequently, he replaced it with a new concept of time based on plausible empirical procedures.
Einstein greatly admired Hume and Mach for their critical philosophies. But another important aspect of scientific thought consisted of a speculative
bottom of p.287


and creative element. On this matter, Einstein once commented to Besso, in 1917, that Mach’s critical approach, by itself, “cannot create anything living, it can only eliminate that which is rotten.”366 In 1952 he elaborated his views on Mach:
“With conviction he had held onto the standpoint, that these concepts, even the most fundamental, receive authorization only from the empirical, that they are in no way logically necessary. He had thereby worked especially to sanitize, as he had made clear, that the most important physical problems are not of a mathematical-deductive kind, but are such that refer to the fundamental concepts. I see his weakness therein, that he more or less believed that science consists of an “ordering” of empirical materials; thereby he had misjudged the freely constructive element in the formation of concepts. He believed, to a certain extent, that theories arise through discovery and not through invention.” 367
Like other physicists, Einstein advanced by proposing and analyzing physical hypotheses. Reportedly, “His imagination conjured up the various approaches. His intuition told him when he was on the right track. It was that way with Relativity—a step at a time.”368 For years he struggled to develop concepts, and combinations of concepts, that would appropriately account for the physical relationships between light and moving bodies. This manipulation of hypotheses and concepts was not restricted univocally by experience. Available experiments did not suffice to simply decide which notions were necessarily true. Theoretical speculations were guided and restricted by experimental knowledge. But experiments alone did not compel the choice of particular concepts. He explained to Solovine: “The concepts can never exactly be derived logically from experience against any objection…. Moral: if one does not sin at all against reason, one generally does not arrive at anything.”369
bottom of p.288


Throughout his life, Einstein emphasized the essentially artificial character of the fundamental elements of physical theories. He argued that creativity consisted of the “free play” of concepts. This license to playfully manipulate, modify, and rearrange concepts was encouraged by Einstein’s childlike approach to physics. He once explained to James Franck that:
“When I asked myself, how it came to be that I in particular found the theory of relativity it seemed to me to lie in this: The normal adult person does not think about space-time problems, he has already done all that there is to think about that in early childhood. By contrast, I was so slow in my development that I first began to wonder about space and time when I was already grown up and hence naturally I penetrated deeper into the problem than a child.”370
In the discussion meetings with his friends, Einstein had cultivated a childlike enthusiasm for fundamental questions. He had also liked to analyze concepts by how they develop in children, according to Solovine.
A key aspect that distinguished Einstein was his great trust on his physical intuition. By intuition he meant precisely knowledge derived by contact with experience, the accumulated understanding of phenomena. Thus in the process of scientific creativity, he argued that “the really valuable factor is intuition!”371 In the end, the irony of his achievement was that even though Einstein began his analyses on the basis of his physical intuitions, he eventually concluded on a solution that was profoundly counterintuitive.
Such factors and others helped Einstein carry out his investigations. And such factors are not unique or original to this case; other scientists throughout history have also employed and benefited from such methods. Yet another aspect that distinguished Einstein from his peers was the consistent obstinacy with which he worked on problems. In an interview, Hans Albert singled out his father’s main distinctive characteristic: “his major trait definitely is his stubbornness,” identifying it as a major reason why Einstein succeeded in the struggle that led to his relativity theory.372 Einstein’s concern
bottom of p.289


over the problems of light and motion became an obsession. A sister of Michele Besso once asked Einstein why Besso had not made comparable achievements:
“Herr Professor,” she asked … “this I really meant to ask you for a long time—why hasn’t Michele made some important discovery in mathematics?”
“But, Frau Bice,” said Einstein, laughing “this is a very good sign. Michele is a humanist, a universal spirit, too interested in too many things to become a monomaniac. Only a monomaniac gets what we commonly refer to as results.”373
bottom of p.290
End of Chapter 6







Friedrich Haller, Director of the Swiss patent office.



Mileva Maric with friends Milana Bota
and Ruzica Drazic in 1899, in Zurich.
Milana Bota referred to Albert Einstein
as “the German, whom I hate.”








Mileva & son in Kleine Schanze park, Bern, in 1904; Kirchenfeld bridge behind them.
Einstein and son, in 1905.





Cartoon drawing of Einstein, by his friend Moritz Solovine, in 1903.

Newspaper advertisement by Einstein, offering private lessons in mathematics and physics, at his home, in 1902.

Einstein’s drawing of his first rented room in Bern, from a letter noting that it had six chairs: “One could hold a meeting here.”
In late 1903, Solovine lived in a rented room above the restaurant “Zum Tramway”
(established in 1899), on 64 Militärstrasse.



The Swiss Federal Office of Intellectual Property was housed in several offices of this building at 6 Speichergasse on the corner of Gernfergasse.


Third-class patent examiner, Einstein,
photographed at the patent office.


The Zytglogge Clock Tower in the center of downtown Bern, at the end of Kramgasse street; built ca. 1218.

By early 1905, Solovine had moved to
an apartment at 16 Gerechtigkeitsgasse.
Einstein, coworkers, friends sometimes met
at the café Bollwerk, near the patent office.


Marcel Grossmann and Albert Einstein.



Albert Einstein in 1896, seventeen years old, at Aarau, Switzerland.



was an important factor in the optics of moving bodies. Also, it constituted an important puzzle in electrodynamics. Since Fizeau’s experiment seemed to show that light did not travel at the same rate in stationary as in moving water, it constituted crucial evidence against Hertz’s theory of electrodynamics, which assumed that the ether in moving media was fully carried along. Consequently, theorists such as Hendrik Lorentz sought to derive the exact algebraic value of the Fresnel coefficient from a theory of electromagnetism applied to bodies in motion.
At some point Einstein became aware of this puzzle. Fizeau’s experiment stood out as a “crucial experiment.”66 It seemed to be “in direct contradiction” to the ether drift experiments, because it seemed to prove that the ether “remains fixed in interstellar space,” being scarcely dragged by moving matter.67 Thus it seemed that a “decision had to be taken,” either in favor of Fizeau’s experiment or the ether-drift experiments, yet this seemed “impossible, for both had operated with unsurpassable accuracy. It was impossible to reconcile both views as they were diametrically opposed.”68 Moreover, the slight “drag” suggested by Fizeau’s experiment seemed inconsistent with another well-known experimental effect: aberration.69 As the Earth orbits the Sun, the light from any star overhead reaches us at an angle, so any telescope must be slightly tilted such that the light may reach its focal point. Any given telescope, unless full of water, did not exhibit any partial dragging of light. Reportedly, Einstein was especially interested in the experiment of the water-filled telescope, carried out by George Biddell Airy in the early 1870s.70 Fresnel had devised his partial drag theory to account for such expected effects.
bottom of p.225
Fizeau’s experiment of 1851. Light enters
on the left, is reflected by a half-silvered
mirror and then is split into two rays that
pass through tubes full of moving water,
the light next reflects from another mirror,
so that it again passes through the moving
water, and reaches an observer. During
the out and back trips, one light ray travels
against the flow of water, the other with it.
If the ether is dragged by the water, the
light moving in the direction of the moving
water should speed up.








Einstein rented a room from a widow at 32 Gerechtigkeitsgasse street.






Walkway arcades of Bern. Albert wrote to Mileva: “There are very old arcades along both sides of the streets, so in the worst rain you can walk from one end of the city to the other almost without getting wet.”


Announcement for the concert of the
“Bohemian String Quartet” playing in
Bern in mid-March 1905.
Hendrik Antoon Lorentz


Excerpt of p.85 of Lorentz’s book of 1895, discussing his concept of “local time.”



Hendrik Antoon Lorentz

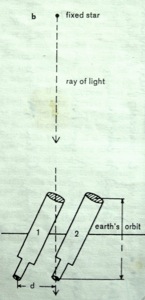
aberration: Earth moves as starlight enters a telescope, therefore the telescope must be tilted so that starlight reaches the focal point of the eyepiece. Light traverses water less quickly than air, but strangely, if the telescope is full of water, the telescope is still focused with the same tilt.



Henri Poincaré, mathematician.
Henri Poincaré in his study.


Henri Poincaré looking serious as usual.

Poincaré’s brief explanation of Lorentz’s “local time” in terms of moving observers who synchronize their moving clocks by exchanging light rays; from his article of 1900, p.272.

Volume in honor of Lorentz, published in 1900, which included Poincaré’s paper.


The “Principle of Relative Motions,” as described in Violle’s book of 1892, p.91.
The “Polybahn” electric cable car
traveling uphill to the Polytechnic.



Professor H. Friedrich Weber
Albert Einstein in Zurich in 1898
A postcard from Einstein, 30 Nov. 1903,
refers to Habicht as “lost member of the
Academy of Sciences Olympia.”
Excerpt from Einstein’s boisterous letter to Habicht, from 18 May 1905.
Zürich waterfront in 1901.

Drude’s Physics of the Aether, 1894.

Second ed. of of Hertz’s book of 1892.
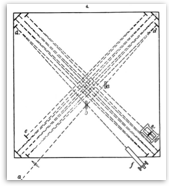



Psychologist Max Wertheimer at around 1914.
Hume’s Treatise of Human Nature, first published in 1739.



Professor Alfred Kleiner, of the University of Zürich.













Einstein’s desk at the patent office.


At the Poly, an international congress
of mathematicians took place in 1897.
Einstein did not pursue mathematics
because he did not sense which topics
were important or promising.
Farm lands around Bern; the Alps in the distance.
Employees at the Swiss patent office.
Prior to Lorentz, George F. FitzGerald
had hypothesized, in 1889, that if bodies
contract as they move through the ether
then that would explain the results of the
Michelson-Morley experiment.

The Fountain of the Ogre, in Bern, shows an ogre eating children from a bag. Sculptor: Hans Gieng, 1544.
The Bern train station, left.






Besso moved to an apartment in this
house, at 15 Schwarzenburgstrasse.





Conrad Habicht rented a room at the house of mathematics professor Johann H. Graf, supervisor of his Ph.D., on 10 Wylerstrasse.

In late 1904, Moritz Solovine lived near the Untertor and Nybegg bridges.



Zürich, Switzerland.

Train station at Casale Monferrato, Italy.


Milan, Italy.



Föppl’s book of 1894, on Maxwell’s theory of electricity.
Föppl discussed the kinematic principle of relative motion and its application to the ether as “perhaps the most important task of natural science of our time.”
In 1831 Faraday discovered that a moving magnet can induce electricity in a wire.


In 1904, Bucherer argued that it was time
to discard the ether concept, to “avoid the
complicated and very artificial hypotheses
of the change of dimensions of bodies...”



View from the left eye: Pearson pursued Mach’s claims about how “outside” things are constructed from perceptions.



Spitalgasse street in Bern.



Kesslergasse street in Bern, with a view of the Münster cathedral.













German translation of Poincaré’s Science and Hypothesis.
How can we know whether lightning bolts at distant places strike at the same time?
The fireplace at Einstein’s apartment.
View from a window in their living room.



Immanuel Kant



Arthur Schopenhauer, 1788-1860:
a role model for messy hair?



The physicist Ernst Mach
Ernst Mach

David Hume


Albert Einstein and Michele Besso

Einstein loved the music of Mozart. While briefly living in Schaffhausen, Switzerland, Einstein and his friend Conrad Habicht played violins in this concert, in December 1901.
Lighting bolts viewed as simultaneous by
a person on the ground, might not seem
simultaneous to someone sitting on a
moving train. Einstein decided that both
observers are correct: Time is relative.



13 May 1905, Einstein and family registered
their new residence, in the southwest side
of Bern, at 28 Besenscheuerweg. It was
later demolished, replaced by this building,
the street is now called Tscharnerstrasse.



In 1904, Besso lived in an apartment in
this building, Falkenhöheweg 15a.



From Jan. to Oct. 1903, Einstein & Maric
lived in an apartment at Tillierstrasse 18.



Einstein rented a room at Archivstrasse 15
in August 1902 until the end of the year.


In early 1905, Besso and his family lived
in an apartment at Zeughausgasse 41,
but they soon moved.


Entrance hallway in Einstein’s
apartment, at night.




Karl Pearson







Einstein and Besso worked on the top floor of
the building that housed the patent office.





Pearson’s critique of the fundamental
but obscure concepts of science.





Immanuel Kant, 1724-1804.



Electric lamp with light
bulb, design patented by
Thomas Edison in 1900.


Unionstrasse 4, Zürich: young Albert Einstein rented a room in this building,
starting on 29 October 1896, from Mrs. Henriette Hägi-Hoffmann.



Klosbachstrasse 87: Einstein rented a
room, from 17 Oct. 1898 to 18 Oct. 1899,
from Mrs. Stephanie Markwalder-Bürgin.


Unionstrasse 4: Einstein moved
back on 19 October 1899.



Left: Dolderstrasse 17, where Albert rented
a room from 11 Oct. 1900 to 17 May 1901,
while Mileva lived just one block away, at
Schönbühlstrasse 15.
Apothecary and drug store on Kramgasse.

Plattenstrasse 50: Mileva Maric rented a
room on the fourth floor of the boarding
house owned by the Engelbrecht family.



The skeptical Scottish philosopher,
David Hume, 1711-1776.